No right angle here
Prove that the internal angle bisectors of a triangle will never be
perpendicular to each other.
Age
14 to 16
Challenge level
Problem
Prove that the internal angle bisectors of a triangle will never be perpendicular to each other.
Student Solutions
This is the solution by Tim from Gravesend Grammar School.
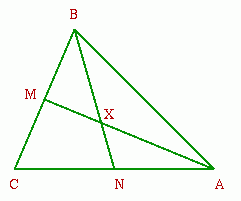
Assume that two of the internal angle bisectors, $AM$ and $BN$, are perpendicular to each other, meeting at $X$, ie $\angle AXB = 90^\circ$.
In triangle $\Delta AXB$, $\angle AXB + \angle BAX + \angle XBA = 180^\circ$
so $\angle BAX + \angle XBA = 90^\circ$.
But $\angle XAC = \angle XAB$ and $\angle ABX = \angle XBC$
so the sum of the angles in the triangle is $2 ( \angle BAX + \angle XBA ) + \angle BCA = 180^\circ + \angle BCA$
so $\angle BCA = 0$, so $ABC$ is not a triangle as it only has two angles,
hence $AM$ and $BN$ are not perpendicular.
Note: What is happening here is that $BC$ is parallel to $AC$.
Image
