A patchwork piece
Problem
I have decided to make another patchwork quilt. The end result should look something like this:

It is a design known as "World without end" and is based on a series of squares like this:
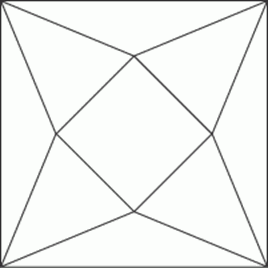
The problem is to make the individual pattern pieces the right size (and shape!).
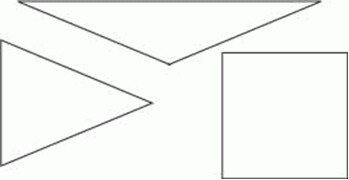
Although it is not exactly obvious this design is based on a regular octagon in a square. These diagrams show the transformations required:
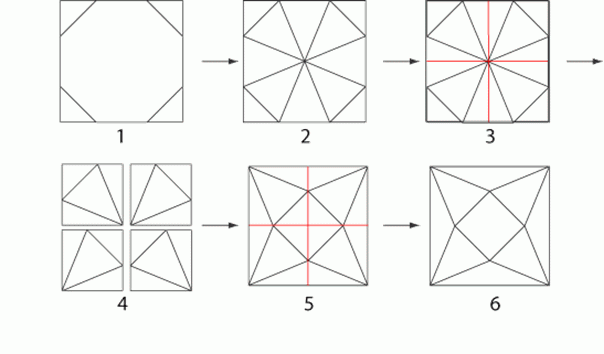
This helps in making the individual pattern pieces. Can you work out how each of the above steps was achieved? Try it with a square piece of paper.
How could you make the pattern if you only have a ruler and a pair of scissors?
What about if you were allowed to use a pair of compasses as well?
Let us know how you achieved each step. You can send in pictures of your final piece, too.
_________________________
Here are the first four squares of the actual quilt:

Getting Started
In the first stage, the octagon you create does not have to be a regular octagon but it is much better if it is.
Which points do the new lines in the second stage join?
The red lines show separate pieces - you might need to do some cutting!
Student Solutions
Step 1
The octagon doesn't need to be regular, so the triangles in the four corners of the square can be any size as long as they are all the same. So, you could start by folding the square into quarters to find the centre, then fold in each of the corners. This would give you a square inside a square, so you need to fold in each corner a second time, up to the first set of folds.An alternative method is to use a compass. Note that all of the vertices of the octagon are an equal distance from the centre, so if you draw a circle and mark the intersections with the square, this will give you 8 vertices.
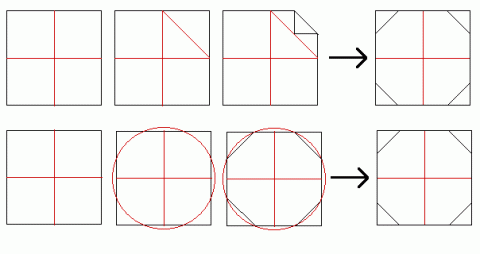
Step 2
The second stage of the pattern is formed by joining together diagonally opposite pairs of vertices.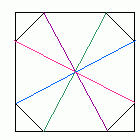
Step 3
Now you can cut the square into four smaller squares using the fold lines that you already have from Step 1. At this point it is easier to see what is going on if you colour in the pieces.
Steps 4,5 and 6
Now that the areas are coloured, can you see that each section has to be rotated individually by 180 °? When you stick the four pieces back together again you are left with the finished pattern!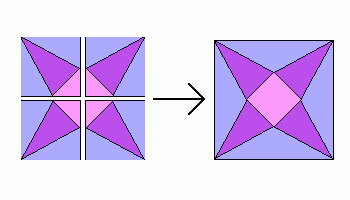
Try it for youself with your own colour scheme, and see if you can find any different ways of making the pattern. You could even come up with a pattern of your own using the same methods!
Teachers' Resources
Why do this problem?
Possible approach
By far, the easiest way to do this construction is to start with an octagon and draw the square round it. Drawing an accurate regular octagon in a set sized square requires mathematical knowledge beyond primary level. Otherwise children can make a design in which the octagon is not regular.
