How far does it move?
Experiment with the interactivity of "rolling" regular polygons, and explore how the different positions of the dot affects the distance it travels at each stage.
Problem
How Far Does it Move? printable sheet
Take a look at the interactivity below which shows regular polygons "rolling" along the horizontal surface.
It leaves a trace of the path of the dot and on the graph it records the distance that the dot travels.
Experiment by positioning the dot at the centre of the polygons, at one of the vertices or at the centre of one of the sides of the polygons and explore how this affects the distance / time graph.
Challenge:
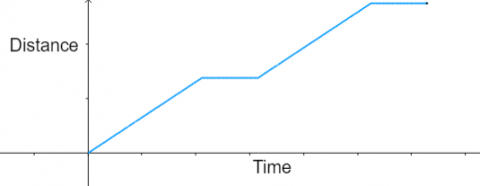
Can you now work out what produced the following distance / time graph?
Can you work out how many sides the polygon had and where the dot was placed?
Try to explain how you worked it out.
Getting Started
Try to approach the problem systematically.
As you go along try to understand why the graph takes the shape that it does:
- by relating it to the rolling polygon and the journey of the dot
- by trying to predict what will happen before you set the polygon rolling
Could the dot have been on the centre of a polygon?
Try for each of the polygons.
Could the dot have been on the centre of the base of a polygon?
Try for each of the polygons.
Could the dot have been on the centre of one of the sloping sides of a polygon?
Try for each of the polygons.
Could the dot have been on the centre of a side opposite the base of a polygon?
Try for each of the polygons.
Could the dot have been on a vertex opposite the base of a polygon?
Try for each of the polygons.
Could the dot have been on a vertex on the base of a polygon?
Try for each of the polygons...
Alternatively...
- try all possible positions of the dot in a triangle,
- and then in a square,
- and then in a pentagon,
- and then in a hexagon...
Student Solutions
This problem clearly got a lot of you thinking! Several of you sent in the correct answer, including Gemma, Rachel, David, Alex, Charlie, Robert, Joel, Jamie, Carys, Soph, Bex, Rhi, Joe, Veronica, Harriet and Elspeth, all from Cowbridge Comprehensive School.
As Azeem of Mason Middle School states:
You have to have a triangle. Also, you must place the dot at the bottom left corner of the triangle.
Some of you worked it out using some systematic thought and trial and error.
Sathya and Michael made a good effort at explaining how they worked this out .
Sathya from Scots College, New Zealand considered whether or not the dot could be placed in the centre of the shapes:
I first checked whether it could have been the centre of the shapes. This is impossible as it always results in a linear graph.
Sathya then went on to consider how many sides the polygon might have:
Then I checked how many segments in the line and I worked out it was a triangle.
Michael from St John Payne School went a little further in exploring where the dot might be placed:
First I knew it had to be on the vertex because there was a part of the graph that was flat.
The only point at which the dot isn't travelling anywhere when the polygon is rolling is on a vertex, because then the dot is always in contact with the floor.
He then looked at the units on the graph to consider which vertex the point would be on:
Hi to 'The Nrich Team'
My 'top' S4 set were this month inspired by your various 'Rolling Polygon' problems.
The class divided into groups of 2 or 3 students. Exact values were the order of the day. The Equilateral triangle and Square were fine though much discussion was needed regarding the final form of the answers. The pentagon proved a lot more challenging with the Golden ratio eventually surfacing.
The groups spent over a week working on this investigation with excitement mounting as the sequence developed. Predictions were made at the stage when the hexagon revealed the number 12 as the 4th term. For the Septagon exact values were not possible so conjectures for the 5th term of the sequence were tested using very accurate calculator work (Sine Rule & Cosine Rule etc).
I feel that the challenge and sheer range of technique required for this investigation has benefited my students immensely. They are aged 14 to 15 and produced work of impressive depth and quality. Thanks for the stimulation that your questions have provided ... keep up the great work. I have attached the write-up produced by David, Nicholas and Robert as it was a superb exposition.... I'm sure you will agree!
We do agree! Many thanks.
Teachers' Resources
Why do this problem?
This problem provides a visual context in which to consider how distance / time graphs represent movement over time. It allows opportunities for learners to discuss and refine their ideas. Asking learners to predict, to justify their predictions and to consider modifying their views can help address misconceptions and improve understanding.
Possible approach
With the dot in the centre, ask the group to predict what the path of the dot will be and what the distance-time graph will look like. Learners could sketch the path and graph in advance, before seeing the polygon roll. Their suggestions could be compared and discussed before making a final joint judgement on the shapes of the path and the graph.
Run the interactivity. Discuss how the graphs related to what learners expected. Confirm understanding by asking what would happen if you changed the number of sides of the polygon.
At this point you might choose to do this card sorting activity. Can the students match each polygon to the path traced by its dot and the distance / time graph of the dot's journey?
When the group feel confident, move them on to more challenging situations by moving the dot to a vertex of a pentagon. Ask similar questions about the path of the dot and the distance-time graph.
Allow plenty of time for discussing/comparing different ideas before running the interactivity for the different polygons or point positions. The pause button is useful to focus on the different stages of the journey and to ask for conjectures about what will follow.
Ask pairs or groups to work on new questions, agreeing and drawing the graph and path together before using the interactivity to confirm their ideas.
Suitable questions are:
What happens if the dot is moved to a different vertex?
What happens if the dot is placed on a vertex of a different polygon?
What happens if the dot is in the middle of a side of a polygon?
The challenge set at the end of the problem asks students to identify the polygon and position of the vertex that would produce the given graph. This creates an opportunity for students to work backwards from the graph and consolidate the ideas developed in the previous work. As well as students identifying which polygon and where the dot is, encourage them to articulate why they know this and how they could convince someone else.
Key questions
- What does the gradient of the graph relate to?
- Why does the dot speed up and slow down at different stages of the "journeys"?
- If we change - (the polygon/position of dot) - what will be the same about the graph and what will be different?
Possible support
Possible extension
Imagine a rectangle, a semicircle or some other shape rolling along.
Follow-up problems concentrating on speed-time graphs and $(x,y)$ position against time are Speeding Up, Slowing Down and Up and Across
