Rod ratios
Problem
Here are three pairs of rods:

The ratio of each of these pairs is 3 : 2.
Can you use the interactivity below to show that this is true?
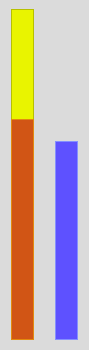
What is the ratio of the pair below? Again, you could use the interactivity to show your thinking.


Getting Started
Can you use the interactivity to help you? You can choose the correct length rods from the interactivity by matching their colours.
Perhaps you could line up white rods alongside the coloured rods?
You could also use the grid lines to help.
Student Solutions
We had just a few solutions sent in for this task.
Monty from the British School in Brussels in Belgium said:
I decided to use the grid mode and count the squares in order to work out the ratios. Here are some of my answers:

Gianna, also from the International School of Brussels in Belgium sent in this good piece of work:

Well done also to Isis (South Island School), Rohaan (Long Bay Primary) and Samantha (The Hamlin School) for completing this task! Samantha explained how she manged to find a pair with the same ratio as the yellow and pink rods:
The pink rod had 4 units and the yellow one had 5 units. This meant that they had a ratio of 4:5. I then needed to find a like ratio. This can be done by multiplying both numbers in the ratio by the same number. In this case the number had to be 2 because the longest rod is 10 units. So the 8 (brown coloured) and the 10 (orange coloured) rods would have the same ratio as the 4 (pink coloured) and
the 5 (yellow coloured).
She also found pairs with the same ratio as the combined yellow+orange to blue:
The ratio of yellow (5) + orange (10) to blue (9) is equal to the ratio 15 to 9. This ratio can be simplified to a 5:3 ratio by dividing both 15 and 9 by 3. Therefore, the yellow and green rods form an equal ratio to that of the yellow + orange to the blue. Another equal ratio would be 10 to 6. Therefore, the dark green
and orange rods also form an equal ratio.
Finally she noted that the rods with the same ratio as 9:6 have already been given!
The ratio of 9:6 can be simplified to the ratio 3:2. The possible combinations with rods for that ratios are shown at the start of the question!
Thank you to all those who sent in their work, thoughts and ideas.
Teachers' Resources
Why do this problem?
Cuisenaire rods are a fantastic resource for investigating ratio. Having the rods as a tool will help children grapple with the quite complex ideas in this task. The rods also provide a visual image which learners might bring to mind at a later date, even when the rods themselves, or the interactivity, are not to hand.Possible approach
If your children are not already familiar with Cuisenaire rods, it is essential to give them time to 'play' with the rods before having a go at this activity. (The way this problem is written assumes some knowledge of the concept of ratio, but Cuisenaire rods could be used to introduce the ideas initially.)You can pose the first part of the task by displaying the image on the interactive whiteboard (IWB) and inviting children to use 'real' rods, or the interactivity on a tablet/computer, to demonstrate why the ratio is 3:2. As they work, you may like to take screenshots of some pairs' arrangements, or warn pairs that you will invite them to the front to re-create their arrangement on the IWB in due course.
During a mini plenary, share different ways of showing how we know that the rod pairs are in the ratio 3:2. Some learners might have used white rods to show the equivalent length of each rod in a pair in terms of whites, then simplified; others might have found a rod which is half the length of the smaller rod in the pair and a third of the length of the longer rod.
As learners begin work on the subsequent parts of the problem, when they are asked to find pairs of rods in a particular ratio, you can encourage them to find all the possible pairs, and to explain how they know they have got them all. The thinking for the final part of the problem has already been done in the first part, but it will be interesting to see whether the class realises this at first!
Key questions
What have you done so far?What happens if we line up [a particular colour] rods against this pair? How might that help?
(Where applicable) Can you find another pair? And another? Can you find all the pairs in that ratio?
