Whirlyball
Whirl a conker around in a horizontal circle on a piece of string.
What is the smallest angular speed with which it can whirl?
Problem
See video [mediaplayer avi - 3.4MB] [quicktime - 0.5MB] [ipod - 1.3MB]
Image

A ball of mass $m$ is attached to a light inextensible string of length $l$. The ball is whirled around so that it moves in a horizontal circle with constant angular speed $\omega$.
Find the angle $\theta$ between the string and the vertical. Show that the angle $\theta$ is given by $\cos^{-1}{g\over l\omega^2}$.
Now increase the angular velocity. What happens to the ball?
What is the smallest angular velocity with which the ball can whirl in a circle on the end of the string in this way?
Getting Started
You can try this with a conker on a string. It is just motion in a
circle with constant angular speed $\omega$. Remember that the
acceleration towards the centre is $r\omega ^2$ where $r$ is the
radius of the circle. Resolving horizontally use 'force = mass
times accelaration'.
Student Solutions
Andrei from Tudor Vianu National College, Bucharest, Romania and Shaun from Nottingham High School both sent in excellent solutons to this problem.
Image
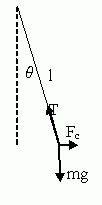
|
After drawing the picture, I observed that the forces
(the gravitational force acting on the ball, the tension in the wire and the
centrifugal force) keep the body in equilibrium. Considering the
centrifugal force, I work in a non-inertial frame of reference,
i.e. in the frame centred on the ball, which is in an
accelerated movement in respect to Earth.
In terms of the vectors we have
|
Resolving horizontally and vertically and using $F=ma$ (where $a$ is the acceleration towards the centre and $T$ is the magnitude of the tension in the string):
Eliminating $T$:
I know that $\cos^{-1}$ is a decreasing function in the interval of interest for the problem. As $\omega$ increases, the angle $\theta$ also increases and the whirling ball rises up, the radius of its circular path also increasing.
The ball can whirl in a circle while $\theta > 0$. So, as I explained above, to find the smallest angular velocity, I have to find the smallest angle. For $\theta \to 0$, ${g\over l\omega^2}\to 1$ and so
The period of this movement is:
Teachers' Resources
An experiment in circular motion using a conker on a string.
The introductory video of 'Shaggy' whirling his conker was created with a program that includes a simulation engine for Newtonian mechanics. We used this engine to animate the conker and its string - so the resulting motion is in a sense 'real'.
Shaggy's hand drives the string. Its motion was defined using trigonometric functions of time applied to the various joints that make up the Shaggy model. In the final 5 seconds the hand moves with exactly half the frequency of the motion which set the conker whirling initially. Its amplitude is however unchanged.
The introductory video of 'Shaggy' whirling his conker was created with a program that includes a simulation engine for Newtonian mechanics. We used this engine to animate the conker and its string - so the resulting motion is in a sense 'real'.
Shaggy's hand drives the string. Its motion was defined using trigonometric functions of time applied to the various joints that make up the Shaggy model. In the final 5 seconds the hand moves with exactly half the frequency of the motion which set the conker whirling initially. Its amplitude is however unchanged.
