Carrying cards
Problem
Carrying Cards printable sheet
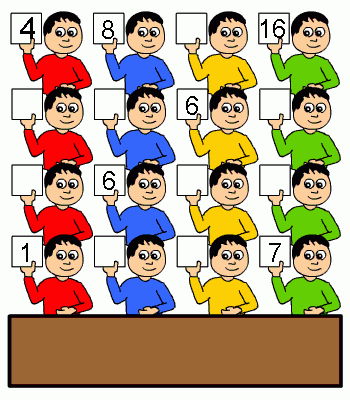
Sixteen children are standing in four rows of four, one behind the other. They are each holding a card with a number on it. In each column, all four children are wearing clothes of the same colour.
|
Red |
Blue |
Yellow |
Green |

The children in yellow each have a number which is double the number of the child in the same row wearing red.
Some of the numbers that the children in red, blue and yellow are holding are missing. What should these numbers be?
How have the numbers of the children in green been worked out? What are the two missing numbers?
If there was another row of four children standing at the back, behind the fourth row, what numbers would they be holding?
Getting Started
To work out the numbers for the children in green, try starting with the first row.
What do you notice about the green numbers? Can you make the green numbers from any of the other numbers?
Does the rule you've found work for the second row of numbers? And the third?
Student Solutions
A Maths Club at Beacon School Amersham wrote:
First we worked out all the missing numbers for the red, blue and yellow children. This was easy because we were told how a number could be made from the number on the card before it in the same row.
Well done! They sent in a table to represent the children's numbers:
| 5 | 9 | 10 | 19 |
| 4 | 8 | 8 | 16 |
| 3 | 7 | 6 | 13 |
| 2 | 6 | 4 | 10 |
| 1 | 5 | 2 | 7 |
Fantastic solutions were also sent in by lots of pupils from Crosshall Junior School; Jun and Colin from the Canadian Academy; Karnan from Stag Lane Middle School; Richard and Jacob from St Thomas More's School and Ellie from West Bridgford Juniors.
Trang from Central Foundation Girls' School, used symbols to help her write out what she needed to do:
Teachers' Resources
Why do this problem?
This problem will give pupils experience of looking for, and explaining, number patterns, and it could lead into algebra. It would also make a good introduction to spreadsheet use.
Possible approach
You could introduce this problem practically in the classroom with children holding small whiteboards, for example. Rather than wearing coloured shirts, the children could have particular coloured pens or they might wear 'bibs' or ribbons usually used for sports matches. Arrange the sixteen children in four rows of four as in the picture and write the given numbers on the appropriate whiteboards. Once the problem has been introduced in this way, the sixteen children can return to their seats and the whole class can discuss the challenge. Learners should be given time to talk to each other in pairs or small groups as well as discussing the problem as a whole class.