Twizzle's Journey
Twizzle, a female giraffe, needs transporting to another zoo. Which
route will give the fastest journey?
Problem
Twizzle, a female giraffe, needs transporting to another zoo as the zoo where she lives has been flooded.
It is important to plan Twizzle's journey very carefully. She must be healthy and calm when she arrives at her new home.
The lorry will need to travel quite slowly, so a short route must be chosen.
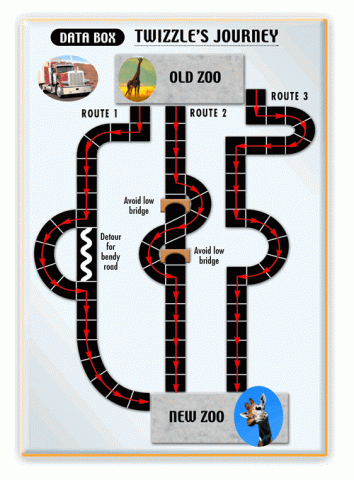
The lorry will be very tall to carry Twizzle, so the route must avoid low bridges.
Bendy roads must be avoided in case Twizzle topples over.
Every three hours, the lorry must stop at the side of the road for 30 minutes so that Twizzle can be checked and given some food and water.
Here is a sketch map of three possible routes:
Each black space on the routes represents 30 minutes of travelling time.
Which route will give the fastest journey?
How long does the journey take?
This problem is taken from "Be a Zoo Vet", one of the Using Maths series published by ticktock Media Ltd. To view their online catalogue, visit the ticktock Media website .
Getting Started
How many black spaces make up each journey?
How many stops would be needed for each journey?
Student Solutions
We had good solutions to this problem from several of you including Mollie who goes to St.Michael's C.of.E Primary School, Adam from Brayton College and Ruth from Swanbourne House School. Ruth said:
First we worked out which route was shortest:Route $1$ has $22$ squares. Each square takes half an hour, so the total time will be $11$ hours.
Route $2$ has $19$ squares, so it will take $9\frac{1}{2}$ hours.
Route $3$ has $25$ squares, so it will take $12\frac{1}{2}$ hours.
So Route $2$ is the shortest. In a journey of $9\frac{1}{2}$ hours, there will need to be three stops. Each stop takes half an hour, so $3$ half hours = $1\frac{1}{2}$ hours.
This means the total journey time will be $9\frac{1}{2}$ hours $+ 1\frac{1}{2}$ hours $= 11$ hours.
Well thought out, Ruth.
Teachers' Resources
Why do this problem?
This problem will help pupils become more confident in calculating with units of time. It is also a practical example of optimisation.
Possible approach
The problem could be introduced by projecting the map onto a screen and children could be given copies of the routes.
Key questions
How long is each route?
Which route is most bendy?
How are you going to decide on the best route?
How many stops would be needed for each journey?