Chain of changes
Arrange the shapes in a line so that you change either colour or shape in the next piece along. Can you find several ways to start with a blue triangle and end with a red circle?
Problem
Here is a set of shapes:
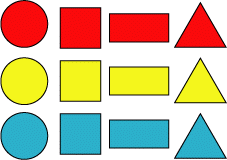
What do you see?
What do you notice?
If possible, talk to somone else about your noticings.
In this problem, we are going to arrange shapes in a line, in a particular order.
You may like to print and cut out a set of shapes from this sheet, or you could try out your ideas using the interactivity below.
Your challenge, as you add a shape to your line, is to change first colour, then shape, then colour again, then shape and so on. (Or first shape then colour...)
For example, if you put the yellow triangle after the blue one and so change the colour, next you must put another yellow piece and so change the shape.
To help you get the idea, below there are two pictures. Each picture shows the first four shapes of a sequence.
Which sequence follows the rule and which does not? How do you know?
| Sequence 1: | Image

... |
| Sequence 2: | Image

... |
Have a go at creating your own sequence of shapes which follows the rule of changing first colour, then shape, then colour... (Or first shape, then colour...)
How many shapes did you use?
Have another go. How many shapes did you use this time?
Is it possible to use more shapes, still following this pattern?
Can you use all the shapes in this way? Why or why not?
Getting Started
Are you going to change the colour or the shape first?
Which shape could you use next?
Can you find another way of doing it?
Can you find another way of doing it that uses more shapes?
Teachers' Resources
Why do this problem?
This problem offers a context in which children will need to look carefully at the properties of each shape. They can be encouraged to refer to the shapes by name and to visualise a possible next shape in the pattern. The problem lends itself to a trial and improvement approach, as well as providing opportunities for mathematical reasoning.
Possible approach
If you have sets of 'Logic Blocks', or similar, then learners would benefit from using them to tackle this challenge. (A full set of 'Logic Blocks' can provide enough for four children, pairs or small groups depending on how the children are working. One group has the large, thick pieces, one the large, thin pieces and so on). Alternatively, you could use sets of the shapes cut out from this sheet (which contains two of each shape). If having practical shapes is not possible, then learners could use the interactivity in pairs on a tablet or computer. The interactivity can provide a useful way of sharing ideas during the lesson, even if practical resources are being used by the children themselves.
You could start with one of the pieces and ask children to describe it. Ask if they can find one which is the same shape but a different colour. In this way, you could build up the idea of the pattern in the problem. To check that learners have grasped the pattern, you could offer the images of the start of two sequences (or make them yourself using the interactivity, or the hands-on shapes) and invite them to say which obeys the 'rule' and which does not. How do they know?
Encourage the children to work in pairs or threes on the main challenge so that they are able to talk through their ideas with each other.
Key questions
Are you going to change the colour or the shape this time?
So, which shape are you going to use next?
Can you find another way of doing it?
Can you do it another way and use more of the shapes?
Can you use all the shapes? Why or why not?
Possible support
Encouraging children to say the sequence out loud (e.g. "red circle, yellow circle, yellow rectangle, blue rectangle...") will help them identify and extend the patterns.
Possible extension
Those who find these tasks straightforward could use a full set of Logic Blocks and so coudl also use the attributes of size and thickness as they create their own patterns.
