Belt
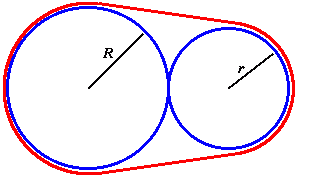
A belt of thin wire, length L, binds together two cylindrical
welding rods, whose radii are R and r, by passing all the way
around them both. Find L in terms of R and r.
Problem
Image
|
A length of thin wire ($L$ ) binds together two
cylindrical welding rods, whose radii are $R$ and $r$ , by passing all
the way around them both.
Find $L$ in terms of $R$ and $r$. |
Getting Started
Where is the belt a tangent to the circles? Draw in radii at these
points.
Student Solutions
Herbert of Sha Tin College, Hong Kong submitted the only correct solution to date which is given below. Can anyone give an alternative solution?
Image

|
$\alpha = \sin^{-1}(R - r)/(R + r)$
$L_1 = R(\pi + 2\alpha)$
$L_4 = r(\pi - 2\alpha)$
$L_2 = L_3 = x$
$x^2 = (R + r)^2 - (R - r)^2$
$x^2 = 4Rr$
$x = 2\sqrt{Rr}$
$L_2 + L_3 = 4\sqrt{Rr}$
|
The total length $L$ is $4\sqrt{Rr} + \pi(R + r) + 2(R -r)\sin^{-1}(R - r)/(R + r).$
