The Dodecahedron
Problem

What is the shortest distance between A and B on the surface?
Getting Started
How do you find the shortest distance between two points on the net of the dodecahedron?
Does the distance on the surface change when the flat net is folded into the shape of the dodecahedron?
Student Solutions
There are two interpretations of this problem, the shortest route between opposite faces of the dodecahedron going across the outside of the solid and the shortest route boring a hole through the middle.
While few tackled this challenging problem, Luke, of Madras College found the shortest distance through the centre. In his solution he used the centres of three spheres: the circumsphere that passes through all the vertices of the dodecahedron, the midsphere touching the midpoints of the edges, and the insphere which touches the opposite faces of the dodecahedron at their centres. Luke's answer is 2.23 approximately. Before you look at Luke's solution here you might like to try to try it for yourself.
On the surface this must be the distance between A and B on the net of the solid.
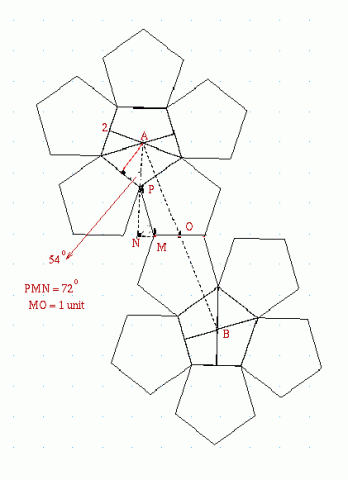
Let the lengths of the edges be 2 units.
AB = 2 x AO
AO = ON + AN
and AN = AP + PN.
Working to 4 decimal places,
AP = 1/cos 54 ° = 1.7015
PN = 2 sin72 ° = 1.9021
NM = 2 cos72 ° = 0.6180
AN = 1/cos54 ° + 2sin72 ° = 3.6034
NO = 2cos72 ° + 1 = 1.6180
AO ² = (1.6180) ² + (3.6034) ²
AO = 3.9499 AB = 7.8999
