Teddy Bear Line-Up
Problem
Lachlan was playing with his bear counters.
He had four blue, then four red followed by four yellow and finally four green bears.
"What are you doing now?" Jenni asked.
"I want to arrange them so that no two bears of the same colour are next to each other", he said.
"That's easy!" cried Jenni.
"Ah, but you have to do it in the least number of moves possible", replied Lachlan.
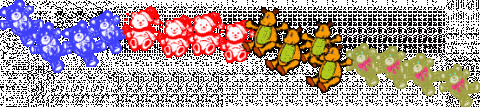
What's the least number of moves you can take to rearrange the bears?
Printable NRICH Roadshow resource.Getting Started
You could start with two bears of each colour to see whether you can find a way.
Have you got a good idea about how to move the bears?
Which bear could you move next?
How do you know how many moves you have made?
How will you remember the moves you have made?
Student Solutions
We received lots and lots of very well-described solutions to this Teddy Bear problem - I'm sorry that we can't mention everyone. It was interesting to see what you called a 'move'. Jessica decided that a move was a 'swap':
Four steps:Start: BBBB RRRR YYYY GGGG
Step 1, Switch the second blue and the second red:
BRBB RBRR YYYY GGGG
Step 2, Switch the fourth blue and the fourth yellow:
BRBY RBRR YYYB GGGG
The fourth red is not used because that would result in two reds being side by side. Switching fourth with fourth makes it easier to keep track of what has been done.
Step 3, Switch the fourth red and the fourth green:
BRBY RBRG YYYB GGGR
Step 4, Switch the second yellow and the second green:
BRBY RBRG YGYB GYGR
The bears are now fully mixed-up. This is not a unique solution.
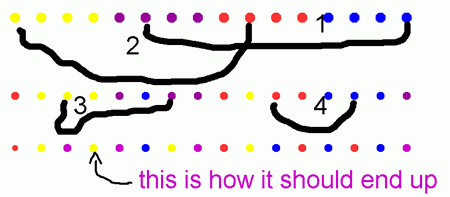
Jessica says this is not a unique solution, meaning that it can be done in four moves, but in different ways. Will, Milly and Emily from Swiss Gardens Primary School agreed with Jessica and sent in this picture of the four moves they made, which are slightly different from Jessica's:
Gemma and Maggie, also from Swiss Gardens, looked at simpler cases with smaller numbers of bears. They said:
We did $2\times2$ which was 1 move also $3\times3$ which was 2 moves.$4\times4$ was 4 moves.
It can be useful to do this to see if you can identify and explain the pattern. I wonder whether you could predict the least number of moves for five lots of five bears?
Jodie thought about a 'move' in a different way and here is what she did:
If you call the blue bears B, the red bears R, the yellow bears Y, and the green bears G, you get:B,B,B,B,R,R,R,R,Y,Y,Y,Y,G,G,G,G
1. Move one of the green bears to separate the first two blue bears:
B,G,B,B,B,R,R,R,R,Y,Y,Y,Y,G,G,G
2. Move one of the yellow bears to separate the second two blue bears:
B,G,B,Y,B,B,R,R,R,R,Y,Y,Y,G,G,G
3. Move one of the red bears to separate the last two blue bears:
B,G,B,Y,B,R,B,R,R,R,Y,Y,Y,G,G,G
4. Move one of the green bears to separate the second two red bears:
B,G,B,Y,B,R,B,R,G,R,R,Y,Y,Y,G,G
5. Move one of the yellow bears to separate the final two red bears:
B,G,B,Y,B,R,B,R,G,R,Y,R,Y,Y,G,G
6. Move one of the green bears to separate the final two yellow bears:
B,G,B,Y,B,R,B,R,G,R,Y,R,Y,G,Y,G
Elijah also used this idea and, like Gemma and Maggie, he investigated simpler cases. He even tried five lots of five bears as well:
Fantastic reasoning, Elijah, thank you for your detailed solution. We can really understand how you were thinking.
We also received the following message from a mum which I couldn't resist including!
My daughter was trying this as we came back from Science Festival today when her little brother found the solution: he picked all the sets of buttons she was using (for counters) and threw them in the air ... as they fell they all scattered ..."Mum! It's just ONE move when HE does it!" Not sure this is what you intended, though...