Top-heavy pyramids
Problem
This problem follows on from More Number Pyramids.
These are top-heavy pyramids. The blue one has $21$ at the apex (top) and the red one has $31$.
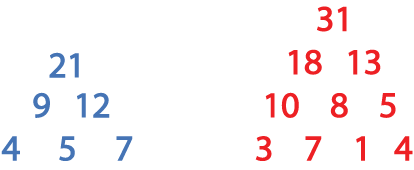
A pair of numbers are added to make the number above that pair.
In the blue top-heavy pyramid whose base is $4$, $5$ and $7$, $4 + 5 = 9$, so $9$ is placed between and above the $4$ and the $5$.
$5 + 7 = 12$ and $9 + 12 = 21$.
Use the numbers in the box below to make the base of a top-heavy pyramid whose top number is $200$.

Getting Started
Order the numbers in a variety of ways. Where a number is placed does make a difference.
Look at the first example given. How many times is the 4 used in
an addition calculation in the pyramid? What about the 5? And the
7?
Try this with the second example too.
Would it help to make up some larger pyramids and then to repeat
this process?
Do you notice a pattern? Could this help?
Student Solutions
Answers with limited explanations of your solutions were sent in by a number of you, including club members from Fen Ditton School, Madras College, Waverley Christian College and King Edward VII School. Trial and improvement (as you can see) is a very acceptable approach. It requires you to look at your findings at each stage of your working and use them, in combination with your mathematical knowledge, to move forward. It is this explanation of why you did what you did next that we want to see. Thank you for your answers.
Correct solutions were received from many
of you including Alex, Stephanie, Charlotte Brant, Rosie, Hannah,
Priscilla and Francesca of The Mount School and Shray of Wilsons
Grammar School. All gave solutions based on the one below which was
sent in by Alex, whose solution gives an excellent insight into
her/his (sorry I am not sure) thinking.
Solution:
I worked out the order of the numbers using just trial and error.
I decided to try the numbers in numerical order first but this did
not work, so I tried putting the smallest number first, then the
largest, then the next smallest, then the next largest
etc...(1,12,3,9,4,8). The apex number was too high so I decided to
try swapping two of the numbers so one of the higher numbers was at
the end and did not have to be added to two different numbers and
only to one.
I decided to use the nine, as my number did not need to go down drastically, which it may have done if I had swapped the 12. So now my sequence was 1,12,3,8,4,9. I had swapped the 9 with the 8. I added all the numbers up and the apex came to 200.
|
200
|
||||||||||
|
103
|
97
|
|||||||||
|
54
|
49
|
48
|
||||||||
|
28
|
26
|
23
|
25
|
|||||||
|
13
|
15
|
11
|
12
|
13
|
||||||
|
1
|
12
|
3
|
8
|
4
|
9
|
I could find no other possible combinations to make the apex total 200.
******
Lucy, Rosie, Hannah, Priscilla and Francesca also offered the following observation, noticed by a number of you.
Using Pascal's triangle we discovered
|
a+3b+3c+d
|
...
|
...
|
||||||||
|
a+2b+c
|
b+2c+d
|
c+2d+e
|
d+2e+f
|
|||||||
|
a+b
|
b+c
|
c+d
|
d+e
|
e+f
|
||||||
|
a
|
b
|
c
|
d
|
e
|
f
|
|||||
We calculated this formula for the top of the pyramid:
a+5b+10c+10d+5e+f
Then using trial and improvement they found the same pyramid as the one above.
****
Andrei of School 205 Bucharest sent in the following. Thank you Andrei.
Let the numbers from the base be a, b,c, d, e and f. Then, the top-heavy pyramid will be:
|
a+5b+10c+10d+5b+e
|
||||||||||
| a+4b+6c+4d+e | b+4c+6d+4e+f | |||||||||
| a+3b+3c+d |
b+3c+3d+e
|
c+3d+3e+f | ||||||||
|
a+2b+c
|
b+2c+d
|
c+2d+e
|
d+2e+f
|
|||||||
|
a+b
|
b+c
|
c+d
|
d+e
|
e+f
|
||||||
|
a
|
b
|
c
|
d
|
e
|
f
|
|||||
So, in top of the pyramid will be:
a + 5b + 10c + 10d + 5e + f = 200
Giving 5 as common factor for the 4 central numbers, I observe that I have the condition that
(a + f) is divisible by 5. The only possibilities are:
(1) a = 1; f = 4
(2) a = 1; f = 9
(3) a = 3; f = 12
(4) a = 8; f = 20
I analyse them separately:
(1) a = 1; f =4
I have: 5(b + 2c + 2d + e) = 195, and (b + 2c + 2d + e) =
39
The possibilities for b and c are 3 and 8, 3 and 9, 3 and 12, 8
and 9, 8 and 12, 9 and 12.
So, the possibilities for the sum 2c + 2d, and b + e are:
c,d |
x=2(c+d) |
39-x |
b,e |
Solution |
|
3, 8
|
22
|
17
|
12, 9
|
-
|
|
3, 9
|
24
|
12, 8
|
-
|
|
|
3, 12
|
30
|
8, 9
|
-
|
|
|
8, 9
|
34
|
5
|
3, 12
|
-
|
|
8, 12
|
40
|
< 0
|
-
|
-
|
|
9, 12
|
42
|
< 0
|
-
|
-
|
(2) a = 1; f = 9
Here (b + 2c + 2d + e) is 38.
c,d |
x=2(c+d) |
38-x |
b,e |
Solution |
|
3, 4
|
14
|
24
|
8, 12
|
-
|
|
3, 8
|
22
|
16
|
4, 12
|
Yes
|
|
3, 12
|
30
|
8
|
4, 8
|
-
|
|
4, 8
|
24
|
14
|
3, 12
|
-
|
|
4, 12
|
32
|
6
|
3, 8
|
-
|
|
8, 12
|
40
|
< 0
|
-
|
-
|
(3) a = 3; f = 12
The sum (b + 2c + 2d + e) is 37, and I have:
c,d |
x=2(c+d) |
38-x |
b,e |
Solution |
|
1, 4
|
10
|
27
|
8, 9
|
-
|
|
1, 8
|
18
|
19
|
4, 9
|
-
|
|
1, 9
|
20
|
17
|
8, 4
|
-
|
|
4, 8
|
12
|
25
|
9, 1
|
-
|
|
4, 9
|
11
|
26
|
8, 1
|
-
|
|
8, 9
|
34
|
3
|
1, 4
|
-
|
(4) a = 8; f = 20
This is the last solution I have to analyse. Here (b + 2c + 2d + e) = 36.
c,d |
x=2(c+d) |
38-x |
b,e |
Solution |
|
1, 3
|
8
|
28
|
4,9
|
-
|
|
1,4
|
10
|
26
|
3,9
|
-
|
|
1, 9
|
20
|
16
|
3, 4
|
-
|
|
3, 4
|
14
|
22
|
1, 9
|
-
|
|
3, 9
|
24
|
12
|
4, 1
|
-
|
|
4, 9
|
26
|
10
|
3, 1
|
-
|
The only solution is:
a, f = 1, 9
b, e = 4, 12
c, d = 3, 8.
| 200 | ||||||||||
| 87 | 113 | |||||||||
| 38 | 49 | 64 | ||||||||
| 20 | 18 | 31 | 33 | |||||||
| 13 | 7 | 11 | 20 | 13 | ||||||
| 9 | 4 | 3 | 8 | 12 | 1 |
Well done to the Red Group from Malvern Parish School who pointed this out.
Teachers' Resources
Why do this problem?
This problem is simple to explain yet involves quite a complicated solution process. This problem will hone skills of addition of two digit numbers whilst challenging the organised mathematical thinking of students. The problem may be done by trial and error or with some appeal to algebra.Possible approach
Key questions
- What is the total for the order $1, 2, 4, 8, 9, 12$? Would we get the same total with a different order? Why?
- What is the largest possible top number for the pyramid?
- What is the smallest possible top number total for the pyramid?
- Which pairs of numbers can be switched without changing the value at the top of the pyramid?
Possible extension
- Are there any other possible combinations of $1, 3, 4, 8, 9, 12$ which lead to the answer?
- What other top numbers are possible? Can you find top numbers which are not possible?
- Are there any other combinations of 6 base numbers which lead to the top number being $200$?
Possible support
Students who struggle with the level of addition might be provided with a simple spreadsheet to do the calculations. They could also be asked simply to work out $5$ pyramids with different numbers to see who can get the largest number or the answer closest to $200$.
