The well known Fibonacci sequence is 1 ,1, 2, 3, 5, 8, 13, 21.... How many Fibonacci sequences can you find containing the number 196 as one of the terms?
These gnomons appear to have more than a passing connection with the Fibonacci sequence. This problem ask you to investigate some of these connections.
Liam's house has a staircase with 12 steps. He can go down the steps one at a time or two at time. In how many different ways can Liam go down the 12 steps?
Beginners to LOGO programming may want to start by working through the FIRST FORWARD series of introductory articles before tackling this problem.
Below are some examples of recursion. I had been trying to replicate the spirals within the head of a sunflower!
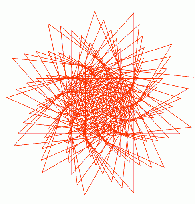
The program used was:
TO SUNF :S :A FD :S RT :A SUNF :S + 1 :A END
You might like to replicate the spirals above by considering suitable values for the variables :S and :A.
The real challenge is to simulate the head of a sunflower with more spirals that are much more tightly packed and look distinctly more 'Fibonacci' in proportion!