Angles inside
Draw some angles inside a rectangle. What do you notice? Can you prove it?
Problem
Draw a rectangle and choose a point inside. Join your point to two adjacent corners, and measure the angles as shown in the GeoGebra applet below.
Try a few examples. What do you notice?
Alison made a conjecture: "The green angle and blue angle add together to give the red angle."
Do your examples support Alison's conjecture?
Can you prove Alison's conjecture?
Can you prove it in more than one way?
What happens if you start with a parallelogram?
Or a trapezium?
What if the point goes outside the rectangle?
Getting Started
You might like to start by exploring the angle relationships in this diagram:
Student Solutions
Lewis from Dartford Grammar School for Boys in the UK, Dang Minh Duc from Renaissance International School Saigon in Vietnam, Alex from Chorlton High School in the UK and Anas all proved Alison's conjecture using the interior angles in a triangle. This is Dang Minh Duc's work:

$b + x = 90^\circ$
g + y = 90$^\circ$
$b + g + y + x = 180^\circ$ (from adding the above equations)
$r + x + y = 180^\circ$ (because angles in a triangle add up to $180^\circ$)
$b + g + y + x = r + y + x$
$b + g = r$
Alternatively, Alex and Lewis expressed $x$ and $y$ in terms of $b$ and $g$:
$x = 90^\circ - b$, $y = 90^\circ - g$, so $r + (90^\circ - b) + (90^\circ - g) = 180^\circ$
so $180^\circ = 180^\circ + r- g-b$, so $r- g-b = 0$, so $r = g + b$.
Leah, Hazel, Kaitlyn, Hollie and Sofia from Ormiston Ilkeston Enterprise Academy, Lewis from Dartford Grammar School for Boys, Alex from Chorlton High School, Baruch from Westminster School, William from The Hurst Community College (all in the UK), Anas, Daniel from Seoul Foreign British School and Thomas from Hollinwood Academy in the UK proved the conjecture using alternate angles. This is Daniel's diagram and Thomas' explanation:

The angle in the top left (green) must be equal to the top portion of the created angle (red angle above the blue line) and the same with the bottom left angle (blue) and the bottom part of the created angle (red angle below the blue line). Thus when you add the two angles up, you get the created angle (red) all by the alternate angles rule!
(You might recognise alternate angles as 'Z' angles.)
William from The Hurst Community College in the UK used a vertical line to form right-angled triangles:

$A\hat{O}C = 90^\circ - O\hat A C$
$E\hat{O}F = 90^\circ - O\hat{E}F$
$E\hat{O}A = 180^\circ - A\hat{O}C-E\hat{O}F$
$\therefore E\hat{O}A=180^\circ - \left(90^\circ - O\hat{A}C\right) - \left(90^\circ-O\hat{E}F\right)$
$E\hat{O}A=O\hat{A}C + O\hat{E}F$
Lewis from Dartford Grammar School for Boys in the UK also proved it using a pentagon:

Sum of interior angles in a pentagon:
$180^\circ (n-2) =$ sum
$180^\circ (5-2) =$ sum
$180^\circ \times3 = 540^\circ$

Each angle between two perpendicular sides, by definition is $90^\circ$. Therefore, we know 2 of the angles in the pentagon are each $90^\circ$.

Reflex on opposite side of point where $r$ is located $= 360^\circ-r$
$540^\circ = 180^\circ + g + b + 360 ^\circ- r$
$0 = g + b - r$
$r = g + b$
Parallelogram and Trapezium
Lewis, Baruch and Anas used the interior angles of a triangle to prove that this also works for parallelograms and trapeziums. The proof is very similar to the proof for rectangles. This is Lewis' working with a diagram added:

1) Co-interior angles sum to $180^\circ
($(g + x) + (b + y)$)
2) The base angles in the triangle sum to $180^\circ- g - b$
($x + y$)
3) The sum of angles in a triangle $= 180^\circ$
4) $180^\circ = 180^\circ - g - b + r$
$0 = r - g - b$
$r = g + b$
William and Anas also proved these cases using alternate angles. These are William's diagrams:

William and also used a method using right-angled triangles:

The yellow and green angles add up to 90$^\circ$
The blue and purple angles add up to 90$^\circ$
So yellow + green + blue + purple = 180$^\circ$
But yellow + red + purple = 180$^\circ$
So green + blue = red.
Baruch thought about what happens when the point is outside the rectangle, and claimed that the alternate angles proof would still work:
If the point goes outside the rectangle, the same theory applies. The “extended” dotted line would still be parallel to AD and CE, so the red angle would still maintain its composition as the sum of the green angle and the blue angle)

However, a closer look at which angles are the alternate angles in this case shows that actually, the red angle is now the difference between the blue and green angles (unless the green angle is now considered 'negative'):

Teachers' Resources
Why do this problem?
This problem provides an opportunity for students to practise measuring angles and review their knowledge of angle relationships in a context which leads to a surprising result. We hope that they will be curious about the unexpected outcome and wish to explore and explain why it happens. The extension questions invite students to explore generalisations of this result to other quadrilaterals or by removing the condition that the chosen point is inside the quadrilateral.
Possible approach
"Draw a rectangle and pick a point somewhere inside the rectangle."
It may be helpful to use squared paper to support students in drawing their chosen rectangle.
"Now join your point to the top left and bottom left corners of your rectangle to create a triangle. It might look something like this."
You could show the picture below or this interactive.
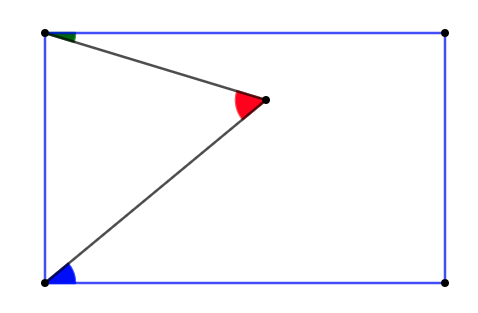
"Measure the angles shown in the diagram and keep a record of your results."
You might like to collect the class results in a table:
| Blue Angle | Green Angle | Red Angle | |
|---|---|---|---|
| 1 | |||
| 2 | |||
| 3 |
"What do you notice about these angles?"
There are plenty of things that could be noticed.
Is the red angle always the largest?
What is the largest value the red angle could have?
Is there a relationship between all the three angles?
You might like to add a new column titled Blue Angle + Green Angle as a prompt if this idea doesn't come up as a noticing.
"It looks like the green angle and blue angle add together to give the red angle.
Is this always true?"
"Can we find a way to prove this conjecture?
Can we prove it in more than one way?"
You may find that due to inaccurate measuring that it is not always exactly the case. This may be a useful time to discuss the limitations of the tools we are using.
Key questions
What do you notice about all the examples?
Is your conjecture always true?
Can you prove it?
Can you prove it in more than one way?
Possible extension
What happens if you start with a parallelogram?
Or a trapezium?
What if the point goes outside the rectangle?
Possible support
To support students who are not familiar with relationships between angles in different contexts you might invite them to explore the relationships between the angles in this diagram.
