You may also like
DOTS Division
Take any pair of two digit numbers x=ab and y=cd where, without loss of generality, ab > cd . Form two 4 digit numbers r=abcd and s=cdab and calculate: {r^2 - s^2} /{x^2 - y^2}.
Sixational
The nth term of a sequence is given by the formula n^3 + 11n . Find the first four terms of the sequence given by this formula and the first term of the sequence which is bigger than one million. Prove that all terms of the sequence are divisible by 6.
Age 14 to 16
Challenge Level
Square Number Surprises printable worksheet
You're probably very familiar with the sequence of Square Numbers: $$1, 4, 9, 16, 25...$$
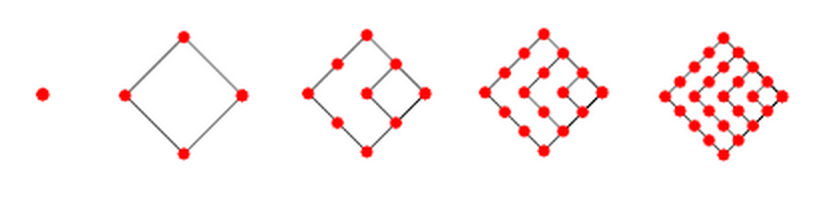
Below are some ideas to explore with square numbers.
For each one, try a few examples and see what you notice.
- Add two consecutive square numbers and then subtract 1
- Square any odd number, then subtract 1
- Multiply two consecutive odd numbers and then add 1
- Multiply two consecutive even numbers and then add 1
Can you explain what you've noticed?
Can you prove that it will always happen?
Can you come up with any Square Number Surprises of your own?
With thanks to Don Steward, whose ideas formed the basis of this problem.
NRICH is part of the family of activities in the Millennium Mathematics Project.