The magic number and the hepta-tree
Problem
The Mathemagician needs to break a dreadful spell! Help him to find the magic number that will do the trick!
Here is the hepta-tree hung with silver balls.
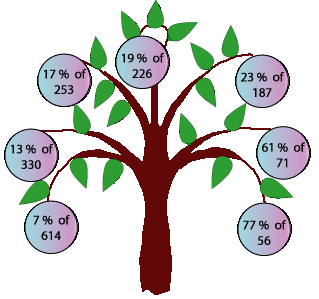
Do you think the balls will have similar values?
Try estimating the amount on each ball.
In order to break the spell, firstly find the exact difference between the largest ball and the smallest ball.
Multiply your result by $244$.
Round this to a whole number and that gives us the MAGIC NUMBER!
Give this number to the Mathemagician and the terrible spell will be broken for ever!
Getting Started
It might be useful to round the percentages up or down to estimate the value of the balls.
You could think of a percentage as a fraction or decimal.
Student Solutions
We received many solutions to this problem, although not so many of you explained exactly how you arrived at your answer. Remember, we're always on the look out for your reasoning too.
Elijah let us know his estimates of the number on each ball:
$7\%$ of $614$ is ~$50$$13\%$ of $330$ is ~$41$
$17\%$ of $253$ is ~$40$
$19\%$ of $226$ is ~$40$
$23\%$ of $187$ is ~$43$
$61\%$ of $71$ is ~$42$
$77\%$ of $56$ is ~$36$
You didn't say how you made these estimations, Elijah. It would be interesting to know ...
'Team Sean', which consisted of Sean, Liz, Briana, Alysha, Amy, Amelia, Chris, Ben, Charlie, Kaden, Matt, Josh, Kate, Stacey, Roy, Mitchell, Dylan, Ed, Emma and Grace gave a detailed explanation of their method for finding the magic number:
Step $1$ -We turned the percentages into fractions and divided the top by the bottom, then we multiplied that answer by the number in the ball. (Alysha)
Example - (Briana) $\frac{23}{100} \times 187 = 43.01$
Step $2$ -
We found the biggest and smallest answers and subtracted the small one from the bigger one. (Dylan)
(Liz) $43.31-42.9 = 0.41$
Step $3$ -
You multiply the answer by $244$ (Josh)
(Sean) $0.41 \times 244 = 100.04$
Step $4$ -
On a number line, from $100.04$, you need $96$ more hundredths to get to $101$ but you only need to go back $4$ hundredths to get to $100$.
Therefore the magic number is $100$ because $100.04$ rounds down to $100$. (Alysha, Charlie and Matt)
We also received good solutions from Adam and Chris who both go to Orchard Primary School, and Callum and Eddie from Stourport Primary. Lucy used a spreadsheet to calculate the percentages rather than a calculator, which works equally well. Well done all of you!
