Fractions in a box
The discs for this game are kept in a flat square box with a square hole for each. Use the information to find out how many discs of each colour there are in the box.
Problem
We have a game which has a number of discs in seven different colours. These are kept in a flat square box with a square hole for each disc. There are $10$ holes in each row and $10$ in each column. So, there would be $100$ discs altogether, except that there is a square booklet which is kept in a corner of the box in place of some of the holes.
We haven't drawn a grid to show all the holes because that would give the answer away!
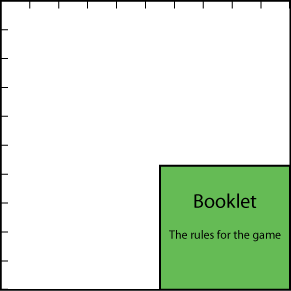
There is a different number of discs of each of the seven colours.
Half ($\frac{1}{2}$ ) of the discs are red, $\frac{1}{4}$ are black and $\frac{1}{12}$ are blue.
One complete row (of $10$ holes) of the box is filled with all the blue and green discs.
One of the shortened rows (that is where the booklet is) is exactly filled with all the orange discs.
Two of the shortened rows are filled with some of the red discs and the rest of the red discs exactly fill a number of complete rows (of $10$) in the box.
There is just one white disc and all the rest are yellow.
How many discs are there altogether?
What fraction of them are orange?
What fraction are green? Yellow? White?
Getting Started
Remember the booklet in the corner of the box is square, so how many holes could it take up?
The whole box, without the space for the booklet, would fit $100$ discs, so the number of discs must be $100$, less the square number taken up by the booklet.
What do you know about the factors of the total number of discs?
Student Solutions
This was a tricky problem. Well done to those of you who had a go. We had some very clearly explained answers. The key was to work out the size of the booklet first.
Rachel, Ol, Jack and Alex from Moretonhampstead Primary said:
Hamish, Rory, Sarah, Jesse and Samuel from Rutherglen Primary also reasoned very clearly and they sent us a picture of the full box which they modelled using cubes:

Sophie and Claire from The Downes School wrote:
$1\times 1$ didn't work because it said that two shortened rows have red discs.
$2\times 2$ didn't work because you need two shortened rows of red and one of orange.
$3\times 3$ didn't work because the total number of discs would be odd and you couldn't halve it. This means all odd numbers didn't work.
$4\times 4$ did work because you had the right amount of shortened rows.
$6 \times 6$ didn't work because you can't divide $64$ by $12$.
$8 \times 8$ didn't work because you need six whole rows.
Emma, Abi, Matthew B and Yuji from Moorfield Junior School; Keshinie and Sharon at Kilvington GGS Victoria, Australia; Gideon from Newberries Primary School and Hannah, Georgia, Patrick; Hana from Bali International School and Matthew from Brighton College Prep School realised that the number left after taking away the booklet must be a multiple of $12$. Keshinie and Sharon describe how they continued from there:
So that made it $84$.
Half of the disks are red so that made the amount of red $42$.
Then it said that a quarter is black so that made it $21$.
Then it said that one twelfth is blue so that made it $7$.
Then it said that one complete row was filled with all of blue and green and the remainder of $10$ if you take away $7$ made it $3$ green.
Then it said that one of the shortened rows is exactly filled with all the orange disks so that makes it $6$.
Then it said that there was only one white disk.
Then we added all the numbers together making $80$ disks so there was a remainder of $4$ which had to be yellow.We divided the $84$ disks by the $6$ orange ones that made it $14$. So the fraction of orange had to be $1$ out of $14$ ($\frac{1}{14}$).
We divided the $84$ disks by the $3$ green disks making the answer $28$. So the fraction of green had to be $\frac{1}{28}$.
We already knew that the fraction of white disk was $\frac{1}{84}$.
We divided the $84$ disks by the $4$ yellow ones making it $21$ so the fraction of yellow had to be $\frac{1}{21}$.
James from the Charter School explained very well how he went about the problem:
Well done too to Harriet and Harah from Greenacre School for Girls, Ruairidh from St Mary's High School, Anne-Marie, Emma, Katherine, Laura from Gorseland Primary and Eulalie and Holly who go to Lympstone Primary School.
The children at Holly Hills Elementary School in the USA had a go at the extension to this problem, from our Teachers' Resources page. Graham and Hayden sent in this picture, imagining what the solution might look like if the booklet measured 6 by 6 instead:
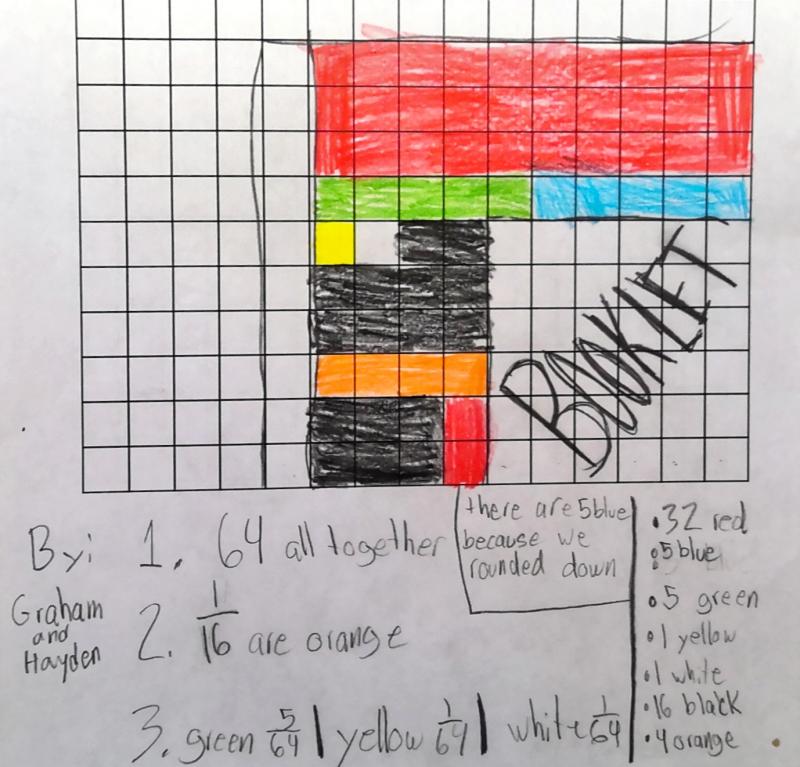
Well done for working these new fractions out! I wonder what fraction of the disks are actually blue in your picture?
Teachers' Resources
Why do this problem?
This problem gives practice in calculating with fractions in a challenging setting. It also requires the use of factors and multiples. While doing the problem learners will need to express a smaller whole number as a fraction of a larger one and find equivalent fractions. This activity will require some estimating and trial and improvement, combined with working systematically.
Possible approach
In the plenary, you could invite the class to look at each other's posters and ask each other questions about the methods used.
Key questions
Possible extension
Learners could find out possible fractions for the differently coloured discs if the square booklet was larger, say $6 \times 6$.
Possible support
Some children my find it helpful for you to structure the approach a little. You could suggest making a list of the possible sizes for the square booklet then working out the number of remaining small square holes for the coloured discs.
