Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Routes 1 and 5
Age 5 to 7
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Routes 1 and 5
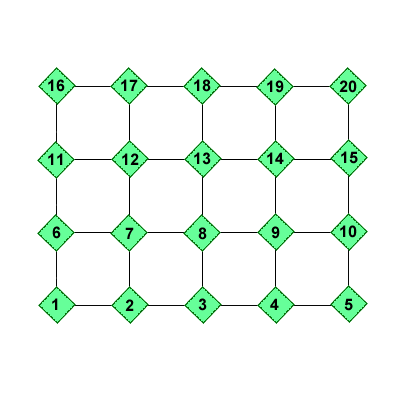
If you look at this grid you will see that when you move in different directions you add or subtract certain numbers. For example, moving one place to the right adds on one, moving up adds 5:
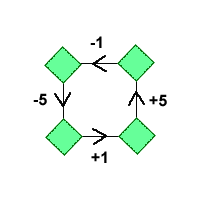
Find your way through the grid starting at 2 and following these operations:
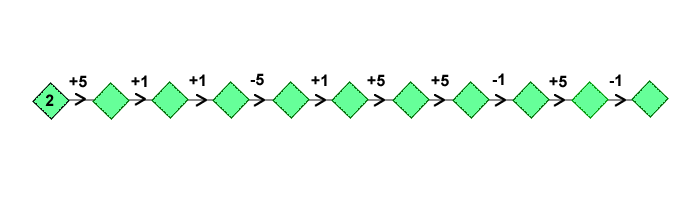 What number do you end on?
What number do you end on?Find another way using these starting and end numbers, and record it in a similar way to the one above.
How can you use what you have done to find the shortest path between these two numbers?
Why do this problem?
At its simplest, this is an exercise in mental addition and subtraction. But this problem also offers opportunities for discussions about inverse operations, a very important concept in mathematical thinking.
Possible approach
Display the grid and ask what the children notice about the numbers. Take all suggestions and make a note of them. Offer the first part of the question and ask the children on which number they end.
Allow some time for them to make up other routes that start on $2$ and end on $18$, and display them where everyone can see them..
Ask for a very long route
Ask for a very short route.
Invite children to explain what they notice is the same about all these routes and what is different.
Listen for reponses that connect the number of $+1$ and $-1$, and $+5$ and $-5$.
Allow some time for the children to make up their own routes for each other, choosing different starting and end points. Suggest that they make up a long route and give it to their partner, who does not look at the grid. The partner works out what the shortest route is (hopefully by matching $+1$ and $-1$, and $+5$ and $-5$) and together they check that both routes work on the grid.
Bring the children together and talk about the way that the numbers match - that they cancel each other out, that they are opposites, and introduce the word inverse if you think it appropriate.
If you have a large playground or school hall you could chalk out the grid on the floor and use the children themselves to play out the routes.
Key questions
What is the shortest route? How do you know?
What would a route look like that started and ended at the same place?
Could you write down a route that got you from the end back to the beginning, without looking at the grid?
Possible extension
You could offer children this sheet of two blank grids to make up their own set of numbers. Have they realised what the underlying structure of the numbers is?
The NNS program Monty uses the idea of hidden grids to reinforce visualisation. If you have internet access, or can download the software onto your system, some pupils will enjoy playing the game against the computer.

