Bugs in the Garden
In Sam and Jill's garden there are two sorts of ladybirds. There are red Seven-Spot ladybirds with $7$ black spots and shiny black Four-Spot ladybirds with $4$ red spots.
Sam and Jill looked at a leaf with three ladybirds on it.
"One Seven-Spot ladybird," said Sam, "and two Four-Spot ones."
"That's $15$ spots altogether!" laughed Jill.
"I wonder if we could find ladybirds whose spots add to other numbers. I know how to do $16$."
"And $14$ is easy too," added Sam.
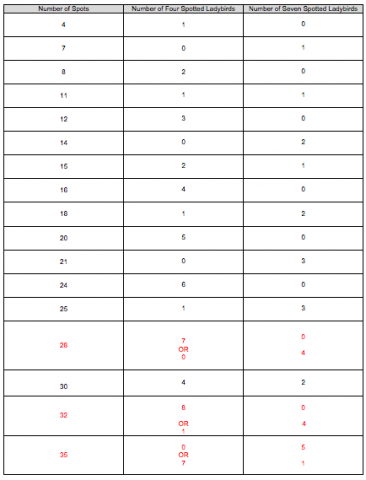
How would you make $16$ and $14$ spots with the Seven-Spot and Four-Spot ladybirds?
What other numbers can you make with adding $4$s and $7$s?
Can you get lots of numbers from say $4$ to $35$?
Are there some numbers you can't get?
Why do this problem?
Bugs in the Garden is a good problem for children to practise addition, subtraction and possibly multiplication. It is a useful context in which to encourage learners to "have a go" and "play" with numbers, but then you can focus on having a system to find all the possible totals and giving reasons why some
can't be made.
Possible approach
It would be good to have the two pictures of the bugs in the problem displayed for the children to see, either printed off or on screen. You could get them started simply by asking how many spots there are altogether on the two, then how many on the picture of the three. You might say that you're picturing some bugs on a leaf whose spots add to $16$ and ask children in pairs to think about
which bugs they are.
Set the class off on the task of finding other possible totals, saying very little more. Wander round the group as they work, looking for different ways of recording and different ways of working. After 5/10 minutes, draw attention to some of these. Some might be writing number sentences, others might be drawing pictures. Some might be starting with a total, others might be starting from a
combination of bugs. It is important to stress that all of these are valid and it depends what each pair finds most helpful. At this stage, remind the children that you'd like them to be sure they have found all the possible totals. How will they know? Invite them to share their ideas which could lead into a discussion of having a system, for example
working in numerical order through the totals, or by using only four-spot bugs first, then only seven-spot, then both.
You could bring their ideas together by asking them to write each different total, and how they made it, on a strip of paper. Gather these strips on the board or a wall and ask the children to arrange them in numerical order. Draw out a list of numbers which haven't been made and ask all pairs to check that they're sure they are impossible. The important point here is for pupils to try to
explain why these totals can't be made. Ask pairs to convince each other and then invite some explanations for all to hear.
Key questions
Which totals have you found so far? Which bugs made these totals?
What totals could we make if there are no four-spot bugs?
What totals could we make if there are no seven-spot bugs?
What totals could we made if there are both four-spot and seven-spot bugs?
How do you know you've made all the totals that are possible?
Why can't we make these totals?
Possible extension
The article,
Opening Out , suggests possible routes for further investigation in general, but this problem is also mentioned specifically. Children could be asked what combination of spots would be needed to be able to generate all the numbers under $30$. Would it be possible to do this without a one-spot bug?
If we couldn't use a one-spot bug, what totals would be possible?
Possible support
Some children might find it useful to have a sheet with consecutive numbers from $1$ to $29$ written on so that they can fill in ways of making each total as they go along. Calculators might be available, or other apparatus to support the arithmetic, for example a hundred square, numberline, Cuisenaire rods or counters/cubes.
This document contains multiple copies of each bug which could be cut into cards. To make the task even more accessible, you could choose and create bugs with $2$ or $3$ spots instead.