Matchsticks
Reasoning about the number of matches needed to build squares that
share their sides.
Problem
| Imagine a matchstick. | Image
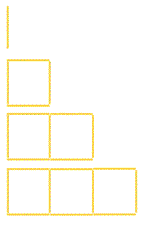
|
| How many more are needed to make a square? How many more need adding to make yet another square along side it? Carry on adding more squares ... |
|
| How many matches have you added? How many matches are there when you have made 10 squares in the row? 20 squares? 50 squares? |
Student Solutions
This solution comes from Henry. Well done!
To make a square, we need three more matchsticks.
To make a second square, we need three more matchsticks.
I noticed that for each square we make we need three matchsticks, in addition to the one we had at the beginning. Therefore to make 10 squares we need a total of 31 matchsticks. For 20 squares we need 61 matchsticks and for 50 squares we need 151.
The general pattern isif $n$ is the number of squares, we use $3n + 1$ matchsticks in total.
