Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Poly Plug Pattern



- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Poly Plug Pattern
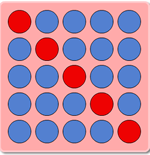
Can you create a pattern on a 5 by 5 board like the one below?

You could print off this board and use counters to create a pattern.
How could you continue the pattern on a large 25 by 25 board like this one?

You could print off this sheet to try out your ideas.
We would love to see pictures of your 5 by 5 pattern and your extended pattern. We would also be keen to hear how you went about extending it.
This activity has been inspired by Doug Williams' Poly Plug resource. You can find out more details, including how to order sets of Poly Plug, on the Mathematics Centre website. However, you do not need sets of Poly Plug to have a go at this activity.
Why do this problem?
Possible approach
Key questions
How could you describe your pattern?
Tell me about what you're doing.
Possible extension
Challenge children to extend their pattern in a different way using the same starting pattern.
Possible support
When using the 25 by 25 grid sheets, some children might find it helpful to have a "window" made out of paper or card which isolates a 5 by 5 section of the grid (like the white box on the image in the problem).

