You may also like
2D-3D
Two circles of equal size intersect and the centre of each circle is on the circumference of the other. What is the area of the intersection? Now imagine that the diagram represents two spheres of equal volume with the centre of each sphere on the surface of the other. What is the volume of intersection?
Power Up
Show without recourse to any calculating aid that 7^{1/2} + 7^{1/3} + 7^{1/4} < 7 and 4^{1/2} + 4^{1/3} + 4^{1/4} > 4 . Sketch the graph of f(x) = x^{1/2} + x^{1/3} + x^{1/4} -x
Fractional Calculus I
You can differentiate and integrate n times but what if n is not a whole number? This generalisation of calculus was introduced and discussed on askNRICH by some school students.
Age 16 to 18
Challenge Level
The following curve turns once. It changes sign twice and the sign of its gradient changes once, giving rise to four distinct regions, as shown in the image below:
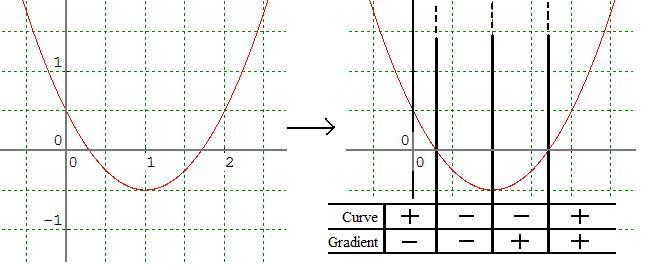
What are the different possibilities of sign combination for a
curve which turns exactly once? How can you be sure you have found
them all? Give a clear explanation.
Which are the different possibilities of sign combination for a curve which turns exactly twice? How can you be sure you have found them all? Give a clear explanation.
Explore other numbers of turns and the curves which give rise to various numbers of regions.
It is not intended that you use algebra; simply experiment with sketches and focus on clear mathematical reasoning.
Further
extensions:
Once you have a good feel for the
setup, you might wish to think about these more tricky or general
questions:
1. Are there any curves which
always have the same sign for the function and gradient?
2. Are there any curves which
always have the opposite sign for the function and gradient?
3. Are there any impossible
combinations of sign?
4. How do zeros of the function
and zeros of the gradient enter into the analysis?
5. Find algebraic expressions
for any functions you use in this problem.
Copyright © 1997 - 2023. University of Cambridge.
All rights reserved.
NRICH is part of the family of activities in the Millennium Mathematics Project.
NRICH is part of the family of activities in the Millennium Mathematics Project.