Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Published 2011
Nriching Mathematics in Haringey
Introduction and Background
In early 2007, Haringey Local Authority and NRICH embarked on a joint project to support the national priority of improving using and applying mathematics, reasoning and creativity$^1$. The idea was to:
-support teachers as they trialed these resources in their own classrooms;
-facilitate shared classroom experiences, thus refining ideas and improving practice;
-strengthen the pedagogy underpinning the new Framework for mathematics so that teachers placed more value on pupils working creatively to solve problems in mathematics;
-establish pedagogic understanding of the importance of using and applying mathematics;
-integrate using and applying into teaching and learning by embedding activities into schemes of work.
Two teachers from each of eleven primary schools within the borough met for the first time in June 2007 to launch the project. The majority were Year 4 teachers, but a few taught Year 5 and others were members of senior management.
During the academic year 2007/8, we met together for one day each half term, working on mathematics as a group, sharing classroom experiences and planning mathematical activities for the coming weeks using the NRICH website.
As the year progressed, several themes emerged as being important, both in terms of classroom practice and professional development. These were:
-Classroom culture;
-Mathematical games;
-Teachers' own mathematics;
-Collaborative continued professional development.
In this article, we aim to outline the key issues listed above by sharing some of the participating teachers' comments and insights.
Emergent Themes
Classroom culture
The atmosphere in my class has changed. The children are intrigued and their perception of maths has changed. I believe NRICH had a lot to do with this.
A new element has been added to maths sessions -discussion/investigation/exploration.
From as early as the second day together, the participating teachers commented on the fact that using NRICH problems had led to (perhaps necessitated?) a new "feel" to the mathematics classroom. Interestingly, the examples the teachers cite often refer to the children's mathematical behaviours but this change could not have taken place without an associated shift in the teachers' values.
During the spring term, to coincide with a unit of work on "calculations and problem solving", one of the teachers at Rhodes Avenue Primary School used four activities with his class during a particular lesson. One of these problems was Sealed Solution:
A set of ten cards, each showing one of the digits from $0$ to $9$, is divided up between five envelopes so that there are two cards in each envelope. The sum of the cards inside it is written on each envelope:

What numbers could be inside the "$8$" envelope?
The children worked in pairs and were asked to share ideas as a form of mini-plenary during each activity. The classroom culture explicitly focused on collaborative work and the teacher emphasised this by encouraging the use of "we" rather than "I'" when pairs were reporting to the class during mini-plenaries. He also used metaphor, "we are in the zone", to describe higher-order thinking skills (HOTS)$^2$, trying to capture how it felt to put a lot of effort into working on a problem. There was an expectation that children's own ideas and thinking would directly contribute to the classroom mathematics. One of the teachers from Earlsmead Primary School, had similar experiences and went as far as to comment that NRICH "encourages peer teaching". Another teacher, from Muswell Hill Primary School, agreed: "children have often taught each other by naturally forming 'mixed-ability' groups or pairs with their friends". As Mason and Johnston-Wilder$^3$ say:
When learners are using their initiative, asking and formulating questions for themselves, helping each other when stuck rather than turning immediately to the teacher, and using their many natural powers in increasingly sophisticated ways, then progression is taking place.
One of the participants from Coldfall Primary School suggested that "lots of interesting questions arise just by the nature of discussing the problem" and that the resources had "helped to broaden the range of questioning that I as a teacher use and that children are used to using". This is worth dwelling on for a moment. This teacher seems to be saying that the NRICH problems directly caused him to reflect on the questions he asks during maths lessons, presumably because he altered his view of what he thinks is important and therefore he has new ideas about what he wants to encourage in the children. These accounts from the teachers represent a significant development in the social interaction between themselves and the children. The development represents a cultural shift away from the teacher being the "authority" or the only mediator between the mathematics and the learner. Children gain confidence because they see that their own contributions are both valued and add to the understanding and development of mathematical problems.
As a result of the teachers beginning to value process rather than outcome, several also talked about their pupils' increased self-assurance:
It has enabled my class to have a more equal access to a maths curriculum because it is not always about getting the "correct answer"$^4$ ... It has given a number of my children a huge confidence boost. The "lower ability" had lots of confidence ... and they contributed much more than had been anticipated. Had we been underestimating them?
It is difficult to summarise in a few words the new classroom culture observed by the teachers. A range of inter-related factors including pedagogy and subject knowledge, linked to the teacher, the pupils and the resources, affects this culture and it certainly does not alter overnight. However, credit should go to all the teachers involved in the project for being open to change and for persevering when sometimes it might have been easier to go back to "more of the same" (MotS), for example with the textbook or worksheets. They did not lose sight of the long-term benefits of what they were doing$^5$.
Mathematical games
Prior to NRICH I saw games as mere fillers. Now they have become a tool to fully explore the children's mathematical thinking.
On our fifth day together, we welcomed Lynne McClure, who led the sessions. Lynne focused on two main ideas during the day: mathematical thinking and games. Over the course of the project to that point, mathematical thinking had become part of our vocabulary and it was extremely useful to tease out what we thought it meant and the implications for classroom practice. However, the focus on games made a huge impression on the participating teachers. Lynne introduced us to many mathematical games, some on the NRICH website and some of her own invention. After playing each, she invited us to reflect on the answers to the following questions:
-What is the mathematical knowledge that is needed to play?
-What is the "value-added" of playing the game?
-Who would this game be for?
-Could you use it to introduce, consolidate or assess?
One of the participants, a newly qualified teacher at South Harringay Junior School, indicated how this session had affected her own practice. On our last day together she said:
I tend to use a lot of the games to try and encourage the "less able" to participate where they don't feel as under pressure. They can take part, they can work in teams or groups ... It has helped certainly the "less able" to be more courageous with maths, to stop worrying, to take risks without worrying they've got to get the answer right the first time round.
She recognises the role that games can play in helping to create the classroom atmosphere described above. Another teacher, from Highgate Primary School, mentioned in particular First Connect Three.
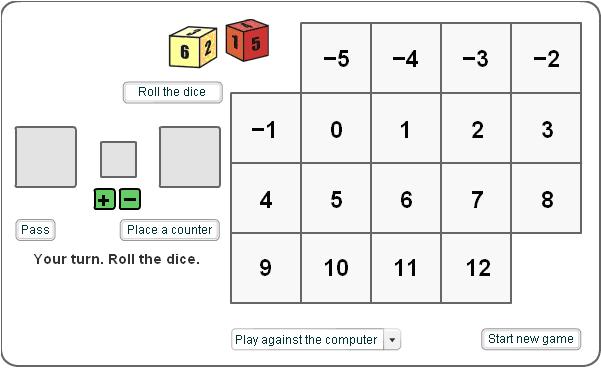
We had played this game altogether, and the teacher from Highgate Primary School subsequently introduced it to his maths group. He said: "It is good for simple calculating and introducing negative numbers but also we've extended it to think about game strategy and making it explicit the idea of what choices they're making". These comments answer three of Lynne's questions and make reference to the higher-order thinking (HOTS) that game-playing strategy can demand.
Teachers' own mathematics

Participating teachers engaging with Stars problem
When we met for the first time in the autumn term, most of the teachers had not used NRICH resources before and were unfamiliar with the website. They represented a wide range in terms of confidence in their own level of mathematics and in their ability to teach the subject. During every session together, we worked on problems or played games ourselves and we felt strongly that this should be a major feature of the year. (It is interesting to note that solving problems and working with colleagues to develop practice are both important elements of the Hampshire Programme "Developing Mathematical Thinking", specifically referred to in Sir Peter Williams' final report (ibid)). In this way, the teachers took on the roles of mathematicians themselves, rather like their pupils would in the classroom. This chance to work on mathematics was a novelty for many, but it proved to be a very positive experience:
I was not really sure what the term "maths investigation" meant. After doing the NRICH course, I have learnt to look at maths from a totally different perspective and not only have I learnt how to investigate maths myself, but [I have] passed it on to my class. I enjoy verbalising strategies I hadn't considered to solve the problems.
We are used to enriching our experiences of English, for example, by reading or going to the theatre. We might listen to CDs or mp3 files and attend concerts if we enjoy music. However, when do we engage in mathematics for pure enjoyment? Working on intriguing problems or playing mathematical games can rekindle a passion for mathematics, or indeed spark off new enthusiasm. In fact, one of the teachers from Rhodes Avenue Primary School, having begun the year not a great lover of mathematics, was keen to share his news at the last session - he was to become Maths Subject Leader from the start of the new term and is hoping to put himself forward as his school's Mathematics Specialist, as recommended by Williams (ibid).
Collaborative continued professional development
At the start of the project, each teacher or pair of teachers decided how to incorporate the activities into their mathematics teaching. Some were working in the context of "setting", others had "mixed-ability" classes. Sometimes an NRICH resource was used as a starter, sometimes as a main activity. A problem might be chosen to introduce a topic or to round one off. However, it was evident that hearing about the ways in which a particular problem or game had been incorporated made other teachers much more inclined to try it too. "The sessions at the PDC have also been great to hear what other teachers have been using in their classrooms and how the children have coped with higher-order thinking skills, mathematical reasoning and pattern finding"
The significance of this chance to share ideas cannot be underestimated. Collaborating in this way encouraged the participating teachers to take risks in their own classrooms, in a way they might not have done without this endorsement from colleagues.
Another key feature of the sessions at the PDC was the allocation of time to devote entirely to planning for maths lessons in the coming half term. The fact that there were two teachers from each school also helped to establish collaboration. The teachers were given printed copies of the Key Stage 2 curriculum mapping document which links NRICH activities to Framework objectives. Armed with their plans for the term ahead and the mapping document, they could begin to tap into appropriate resources for their classes. What was fantastic to see was the way that, gradually speaking, the mapping document was used less and less. Instead, as they became more familiar with the search facilities on the website, the teachers looked for activities themselves rather than relying on the mapping's recommendations. This flexible use of the mapping document was intimately linked to the sharing of practice. If one teacher reported success with a particular problem for a particular purpose, others tried it too, without considering whether it featured in the mapping. This is exactly how the NRICH team intended the mapping to be used - as a way in to the site - and so it is wonderful to have seen this process in action.
It was clear that the time allowed for planning together in this way was welcomed. "It's made planning maths more enjoyable!" What more can we say?!
Long-term Impact
In all that we have written above, it is obvious that we believe there have been many positive outcomes to this project. Headteachers of participating schools have commented on noticeable developments in classroom practice and improvements in the attainment of some children. In one "low-attaining" "set", many children gained two levels of progress during the year of the project. However, we would consider it a failure if it had no long-term impact on the participating teachers and schools. We particularly asked the participating teachers to describe how the project would affect future teaching and learning in their schools, and there were very encouraging signs. Of course, given they had all added some NRICH activities to their existing Maths plans, at the very least, we might expect those that were due to stay in the same year group to be able to use the resources again in similar ways. Happily, many were aiming to go beyond this.
Teachers who knew they would be working in a different year group or with new colleagues in the coming academic year, were sure they would use NRICH again and spread the word. They all committed to this and senior leadership was involved. For example, at Earlsmead Primary School, the head teacher wanted to encourage all teachers in the school to use the NRICH mapping document when they are planning. Rhodes Avenue seemed to go a step further and, at a staff meeting, it was decided that NRICH activities would be embedded throughout the school as the teachers believe they have had a real impact on children's attainment levels.
Concluding Remarks
We are very grateful to Haringey LA for funding this project and for acknowledging its success by securing funding for a further joint venture for the year 2008/9.
Michael and Liz would like to thank the participating teachers and Senior Management Team from all the schools involved:
Bruce Grove Primary School
Coldfall Primary School
Earlsmead Primary School
Highgate Primary School
Muswell Hill Primary School
Rhodes Avenue Primary School
Rokesly Junior School
South Harringay Junior School
St. Gilda's RC Junior School
St. Ignatius' RC Primary School
Stroud Green Primary School
Haringey and NRICH continued to work together after this project reached its conclusion. Two Year 5 teachers from each of twelve different schools came together for six face-to-face days during the 2008-9 academic year to further develop the work begun in this project. And in 2009/10, Haringey and NRICH worked with two teachers from another twelve primary schools in the borough.
References
($1$) See for example:
The ability to pose and answer questions and to solve problems lies at the heart of mathematics and children often find these more creative aspects of mathematics difficult.
Raising standards in mathematics - achieving children's targets, DfES, Ref. 1075-2004
... the development of investigative approaches that characterise using and applying mathematics remains weaker than other aspects of the mathematics curriculum.
The Annual Report of Her Majesty's Chief Inspector of Schools 2005/6, Ofsted
The nature of teaching and assessment, as well as the interpretation of the mathematics curriculum, often combine to leave pupils ill equipped to use and apply mathematics. Pupils rarely investigate open-ended problems which might offer them opportunities to choose which approach to adopt or to reason and generalise. Most lessons do not emphasise mathematical talk
enough; as a result, pupils struggle to express and develop their thinking.
Mathematics: understanding the score, Ofsted, 2008
($2$) Year 6 and know it all...? McClure, L., Primary Mathematics, Summer 2007
and
Nutshells, interactive professional development resources, originally devised for NAGTY
($3$) Designing and Using Mathematical Tasks Mason, J. and Johnston-Wilder, S. (2006) Tarquin Publications. (This publication is one of the readers for the OU MA (Education) module ME 825, Researching Mathematics Learning.)
($4$) Interestingly, what this teacher says here resonates strongly with research findings into effective pedagogy, quoted in the Williams Review: "focusing on the mathematics rather than "getting the right answer..." Independent Review of Mathematics Teaching in Early Years Settings and Primary Schools, Final Report, Sir Peter Williams, June 2008, DCFS
($5$) The long term benefits of effective CPD is discussed in the Williams Review, which also refers to a report by ACME: Ensuring effective Continuing Professional Development for teachers of mathematics in primary schools, ACME, 2006.

