Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Age 11 to 18
Published 2008 Revised 2021
Geometry: A History from Practice to Abstraction
The story of the development of non-Euclidean geometry is one of the classic adventures in the history of mathematics. The Greek mathematicians changed geometry from what was an important useful, everyday, practical tool to an intellectual enterprise for the unification of known mathematics on a theoretical basis . Euclid's 13 books of the Elements contain the foundations of number theory, ratio and proportion as well as plane and solid geometry. Later Greek scholars like Apollonius, Eratosthenes Archimedes, Diophantus and Hiero developed more ideas about theory and practical applications that were handed on to the Arabs.
In their turn, Arab mathematicians took on the Greek mathematics, but were also importantly influenced by mathematics and astronomy coming from India, and even as far as China. For the Arabs, practical astronomy was an important enterprise. Their religion required them to find the exact location of Mecca, so that the direction of Mecca from any part of their world, could be easily found. To enable these calculations, they invented most of the basic techniques of trigonometry, and developed Spherical Geometry. Apart from their practical work, scholars like Al-Khowarizmi and Omar Khayyam made major contributions to the 'pure' mathematics of geometry and algebra. There was, in fact, quite a lot of contact with Christian and Jewish scholars, exchanging ideas and translating important works, in spite of periods of conflict and pestilence. The so-called 'dark ages' in Europe were not so dark as some have made out.
What is interesting in the story, is that for a long time, the different developments in geometry described in this article were motivated by purely practical aims; astronomy, pictorial representation, and architecture. Each development had its own theoretical basis and had relied on previous knowledge of earlier mathematics and technology. For example, in the 10/11th century the optics of Alhazen (Ibn al Haytham) was developed in an almost modern scientific atmosphere that involved "criticising premises and exercising caution in drawing conclusions and taking care in all that we judge and criticise that we seek the truth and not be swayed by opinions".
However, the unification of all these ideas did not even begin to happen until the beginning of the 20th century. There were many reasons, but for us as teachers, what we can note particularly is the reluctance to take on new ideas, to be open to the 'what if' possibilities, and the gradual abstraction of geometry and algebra in the 19th century which brought about the unification of large parts of mathematics based on ideas of structure .
Now we can see the different branches of mathematics as being parts of a whole, but importantly, no part is really separate from the others. We describe geometry in algebraic terms (due to people like Descartes and Galois), but we also see algebra as geometric patterns (from the work of Poincare and Mandelbrot). There are always opportunities in our teaching to make links between 'topics' and break down the barriers that curriculum and text-book chapters have created in the cause of 'efficiency'.
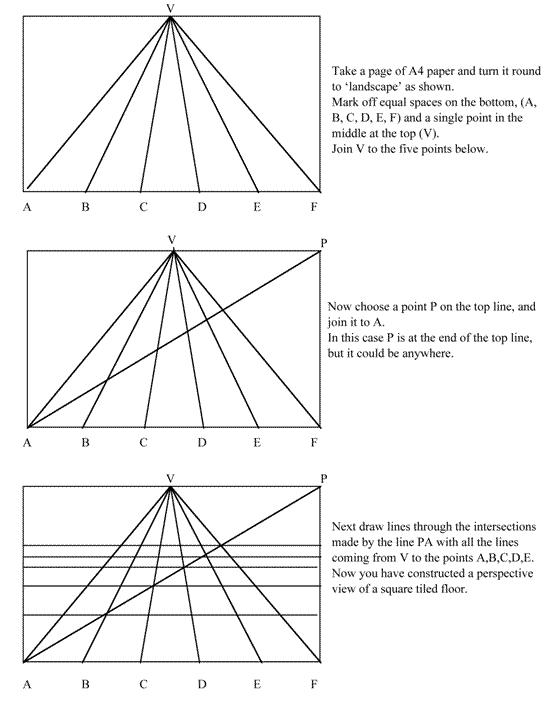
Perspective Explorations
An excellent book on the subject is by Judith Field,
The Invention of Infinity:
Mathematics and Art in the Renaissance (OUP 1997). The
picture below is a variation of Piero's 'pavement' technique. You
can use the picture and the questions to work with pupils on
developing ideas on similarity and perspective. A copy of this
image can be found in this Word document.
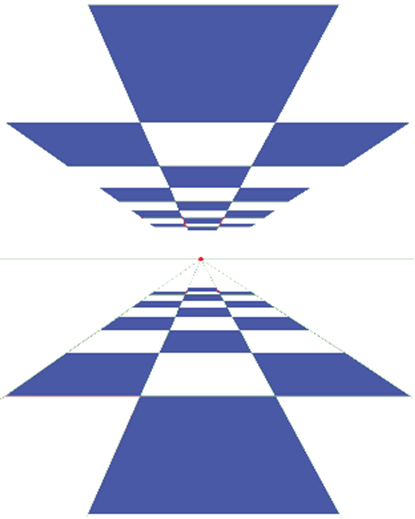
How would you describe this picture?
There are a number of similar shapes here. Can you find them
and explain why they are similar? How would you describe the
relation between the shapes?
Can you extend the picture and draw another row of shapes on
the bottom? Start in the bottom right hand corner and draw a
diagonal of one of the shapes.
Extend this line across the picture. What do you notice?
Here is another exercise: