Problem | Teachers' Notes | Hint | Solution | Submit a solution | Printable page |

This problem extends the introductory Bridge Builder problem. You will probably first need to attempt that problem .

Our bridge building engineer wishes to consider the problem of making a longer bridge with n segments along the base


For a bridge of even length, a single weight 2W is to be hung from the centre. For a bridge of odd length, two weights W will be hung from either side of the central strut, as in the following diagrams:

Part 1) Which lengths of bridge can be created such that there is no net force on any of the pin joints? Try to solve this problem using only vector methods.

Part 2) If a bridge cannot be created with no net forces at all joints, can we say which pin joints necessarily have a non-zero net force? How could you alter the design of the bridges which experience a net force at a joint to remove this force (without changing the length of the bridge)?

Part 3) Which struts do you think will be under most tension/compression. Perform a calculation to see if you are correct.

Part 4) Investigate the effects of adding an extra layer of triangles to the top of the bridge or changing the configuration of the supports. Which configurations can you make which can be created with zero net force at all of the joints?


For inspiration in creating these structures you might want to look at the Forth Bridge, in Scotland (see, for example the Forth Bridge official website http://www.forthbridges.org.uk/railbridgemain.htm ) , which is built using the following basic framework structure:


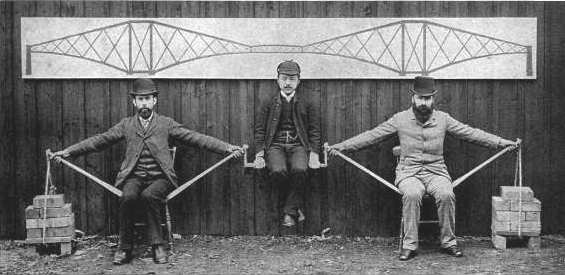
In 1887 the designer of the Forth Bridge, Benjamin Baker, modelled the key principles involved in the bridge's design using two piles of bricks, two ropes, four wooden poles and three people and a seat. The man in the centre is very easily supported as his weight is distibuted throughout the structure.

Extension: The Forth Bridge uses a criss-cross design. Can you work out how the forces are distributed amongst all of its supports? Which parts of the bridge experience the greatest tensions and compressions?

Resultants and components of forces. Newton's laws of motion. Angular Motion. Animations. Short problems. Moment of a force. Rigid Bodies. Energy. Circular motion. Centre of mass. Forces.