Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Rod Measures
Age 7 to 11
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Rod Measures
Using 3 rods of integer lengths, none longer than 10 units and not using any rod more than once, you can measure all the lengths in whole units from 1 to 10 units. How many ways can you do this?
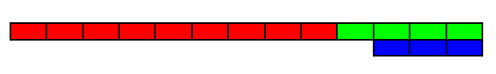
For example with rods of lengths $3, 4, $ and $9$ the measurements are:
$4-3,$ $9-4-3,$ $3,$ $4,$ $9-3,$ $9-4,$ $3+4,$ $9+3-4,$ $9,$ $9+4-3,$
Using 3 rods of ANY integer lengths, what is the greatest length N for which you can measure all lengths from 1 to N units inclusive? Can you beat 10 units? Can you beat the highest value of N submitted to date?
Why do this problem?
This excellent problem is so very good for number awareness, and reinforcement of addition and subtraction rules.
Possible approach
Starting off in a very practical way with suitable rods would be ideal in many circumstances.
Key questions
How did you get to this solution?
I see you've not got a (suppose - 9 when using 2,3 & 5) can you explain that?
Possible extension
What about four rods?
Which combinations work/do not work and why?

