Slippery Slopes

This resource is from Underground Mathematics.
 

Here are the graphs of four functions. The equations of the graphs are

$$y=f(x) \quad y=f(x)-8 \quad y=3f(x) \quad \text{and} \quad y=3f(x)+8.$$

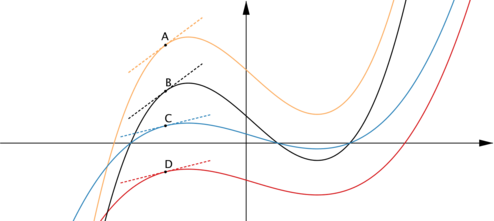
• The $x$-coordinates of $A$, $B$, $C$ and $D$ are all the same.  What can you deduce about the gradients of the curves at $A$, $B$, $C$ and $D$?
• The gradient of the tangent at $D$ is $\tfrac{1}{4}$. What are the gradients of the tangents at $A$, $B$ and $C$?

Here are the graphs of another four functions. The equations of these graphs are $$y=f(x)\quad y=f(x+20)-8 \quad y=3f(x-25) \quad \text{and} \quad y=-3f(x)+10.$$

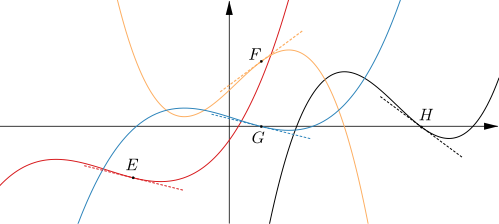
• The $x$-coordinates of points $E$, $F$, $G$ and $H$ are $-15$, $5$, $5$ and $30$ respectively. What can you deduce about these points?
• The gradient of the tangent at $E$ is $-\tfrac{1}{4}.$ What are the gradients of the tangents at $F$, $G$ and $H$?