World of Tan 25 - Pentominoes
Can you fit the tangram pieces into the outlines of the people?
Age
7 to 11
Challenge level
Problem
This activity follows on from World of Tan 24 - Clocks.
School is over for the week. In the yard, the workers are getting ready to go home. Little Fung and Little Ming are getting in the way as usual, but their puzzle has captured the attention of the supervisor, Chi Wing. They are making shapes using five small squares on a grid.
Image
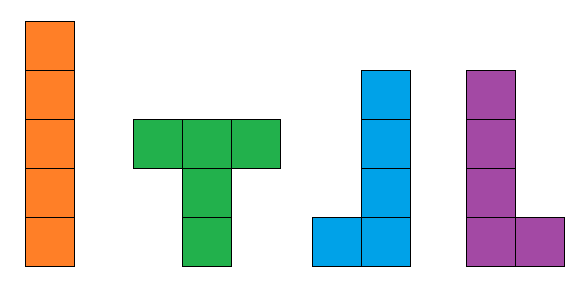
Little Ming: The teacher says there are eighteen different shapes!
Chi Wing: That might be true, but I don't believe it.
Little Fung: If the squares are joined together properly there are eighteen - she said so.
Chi Wing: Have you found them?
Little Ming and Little Fung pause and look at each other.
Chi Wing: No you haven't! I don't think you have anywhere near eighteen solutions. You two never work systematically at anything.
Little Ming: We do have seven though...
Chi Wing: Listen, what exactly were you asked to do? Little Fung, please can you explain.
Little Fung: Each shape is made up of five squares.
Little Ming: They always have to be five squares!
Chi Wing: Let Little Fung finish explaining. Little Fung, carry on please.
Little Fung: The squares have to be joined in a special way, edge to edge.
Little Ming: Yes, not corner to corner or...
Chi Wing: Little Ming, you must be quiet and let Little Fung finish.
Little Fung: The edges must be completely touching each other.
Chi Wing: Okay, I think I understand. Can you both show me what you've done so far?
Little Ming and Little Fung show Chi Wing their shapes on the squared paper.
Chi Wing: So if we move them around systematically we should be able to find the eighteen different ones.
Little Fung: Yes... but we can only find seven...
Chi Wing: Let's start again at the beginning with all the squares in a line.
Little Ming: Okay, let's begin by moving one square at a time.
Chi Wing: So which square should we start with?
Little Fung: Does it matter?
Little Ming: Yes, we need to be systematic like Chi Wing was saying, so that we can find all the possibilities.
Chi Wing: So instead of bickering, which square should we start with?
In the meantime, complete the silhouettes of Chi Wing studying the problem, then working systematically.
Extra activities:
- Find the eighteen shapes that Little Ming and Little Fung are searching for.
- How many of these shapes could be folded up to make boxes?
- Which of these shapes are reflections of each other?
- What if Little Ming and Little Fung had used six squares instead of five? How many shapes would they be able to make? How many of these shapes could be folded up to make boxes with lids?
Teachers' Resources
Why do this problem?
This problem is an engaging context in which pupils can consolidate their knowledge of the properties of squares, triangles and parallelograms. By attempting this activity, children will be putting into practise their visualising skills, making guesses about where the different shapes might go before trying out their ideas. When combining the shapes to make the tangram, pupils will use their understanding of translations, reflections and rotations to decide how to transform each shape. There are also links between tangrams and fractions, and children can be encouraged to work out what fraction of the whole square is represented by each smaller shape.Possible approach
Read this story with the whole class and look at the tangram as a group. Ask pupils to suggest where a shape might go. What transformation would be needed to move the shape into that position?When pupils are solving the tangram, they would benefit from working in pairs with a tablet or a printed copy of the shapes to cut out and move around. Working together will lead to rich discussions about the possible options for where each shape can go. When the children have solved the tangram, they can have a go at the extra activities.
At the end of the lesson, bring all of the pupils together and model the solution on the whiteboard. How does each shape need to be transformed? What fraction of the whole picture is each shape?
Key questions
What could you put with this piece to make a square?Are all of the pieces different?
What's the smallest square you can make?
What has to go in that space? How do you know?