How much?
Problem
Dan bought a packet of crisps and an ice cream.
The cost of both of them together is in one of the boxes below.
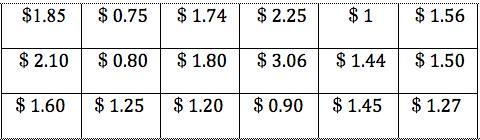
Use these clues to find out how much he paid:
1. You need more than three coins to make this amount.
2. There would be change from the most valuable coin.
3. The crisps cost more than $0.50.
4. The ice cream costs exactly twice as much as the crisps.
If you are using pounds instead of dollars then go to 
Getting Started
It might help to make a list of all the coins first, just to remind yourself.
Are there any amounts which you can definitely rule out using some of the clues?
Student Solutions
Tim from Ysgol Uwchradd Tywyn wrote:
I ruled out anything under £1 because it has got to be more than 50p. Anything doubled over 50p makes more than £1.I ruled out anything over £2 because you get change from the biggest coin, being £2.
Then I ruled out anything ending in anything apart from 0. Because you can't use coppers.
I was left with: £1.80 £1.60 £1.50 £1.20
I came to the answer of £1.80 because 60p doubled is £1.20, add them added together is £1.80. Meaning the ice cream is £1.20 and the crisps are 60p.
Tom from the same school as Tim went about it in a slightly different way:
The crisps would cost 60p and the ice cream would cost £1.20 making my solution £1.80I worked this out by discounting anything below £1.65 based on minimum amount of crisps and double amount of ice-cream and the no copper coins. 75p, 80p, £1.25, £1.20, 90p, £1.00, £1.44, £1.45, l.56, £1.50 and £1.27
I discounted anything that wasn't in the 5x table because of the no copper coins rule. £3.06 and £1.74
I also discounted anything that wasnt divisible by 3 into a number in the 5x table because the ice-cream is twice as much as the crisps and the copper coin rule. £1.85 and £1.60
Finally I discounted anything that could be paid with 3 coins or less. £2.10 and £2.25
Leaving only £1.80
Here is another solution from Hayden from Davenies School who used the clues in a slightly different order:
I think the answer is £1.80.The most valuable coin is £2 so I crossed out answers of £2 or more.
Then I crossed out any answers that needed copper coins.
Then I worked out that the crisps and ice cream had to cost more than £1.50 so I crossed more out.
I then crossed out any amount that could be paid with fewer than four coins.
This left me with two possible answers: £1.80 or £1.85.
As the ice cream costs exactly twice as much as the crisps, the answer is £1.80.
Morgan and Daniel from Greystoke Primary had another way again:
Using the clue that you will need more than three coins we eliminated 75p, £2.25 £1, £2.10, 80p, £1.50, £1.60, £1.25, £1.20 and 90p.After that we moved on to the second clue - 'There must be change from the most valuable coin'! The most valuable coin is £2 so we could rule out £3.06
Then we moved onto the clues 'The crisps cost more than 50p' and 'The Ice Cream will cost double what the crisps cost!' Therefore we could rule out totals under £1.50 ( £1.44, £1.45 and £1.27)
This left us with four options £1.56, £1.74, £1.85 and £1.80 We could rule out £1.50 and £1.74 using the 'You could pay without using copper coins clue'.
Now we had £1.85 and £1.80 remaining. We discarded the £1.85 because you can't have a total and a total half of it!
£1.80 is the solution!
Finally, Daniel and Connall sent in their solution in the from of a table where they give reasons for eliminating all the other amounts. You can see their work in this Word document . This is very easy to understand, thank you boys.
Thank you, too, to everyone else who sent in a solution agreeing with the answer of £1.80.
(When this problem was first published on the site, we made a mistake. We've now corrected it but would still like to thank all those of you who wrote to point out our error. Children at Much Wenlock Primary School, Hotwells Primary School, Downsview School, King Henry VIII Abergavenny, Brocks Hill Primary School, Eastwood Comprehensive, Rebecca at Gateway Primary School and Benjamin at Holmead Middle School all explained that in fact it was impossible to solve.)
Teachers' Resources
Why do this problem?
Possible approach
Key questions
Possible extension
Possible support
