 Links to the NRICH website Home page
Links to the NRICH website Home page

30 April (Primary), 1 May (Secondary)
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Of All the Areas



Of All the Areas printable sheet
You may wish to print off some isometric paper or use the isometric dotty grid environment for this problem.
This problem follows on from Isometric Areas and More Isometric Areas.
When working on an isometric grid, we can measure areas in terms of equilateral triangles instead of squares.
Here are some equilateral triangles.
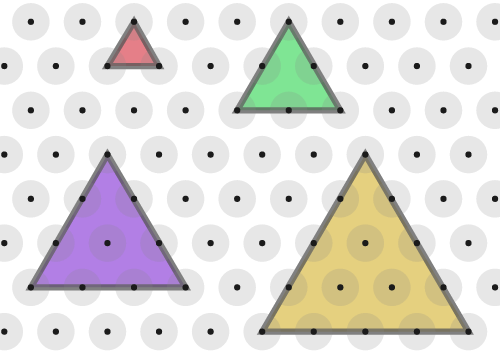
If the area of the smallest triangle is 1 unit, what are the areas of the other triangles?
Can you see a relationship between the area and the length of the base of each triangle?
Will the pattern continue?
Can you explain why?
All the triangles in the first image had horizontal bases, but it is also possible to draw "tilted" equilateral triangles.
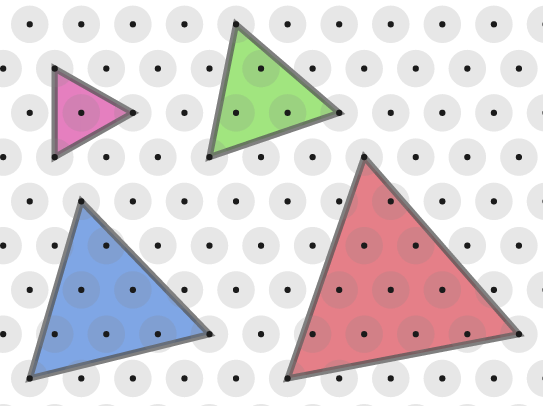
These triangles all have a "tilt" of 1.
Can you convince yourself that they are equilateral?
Can you find their areas?
Take a look at the hint for ideas on how to get started.
Can you find a rule to work out the area of any equilateral triangle with a "tilt" of 1?
Can you explain why your rule works?
What about areas of triangles with a "tilt" of 2?
What about areas of triangles with other "tilts"?