Copyright © University of Cambridge. All rights reserved.
'Ribbon Squares' printed from https://nrich.maths.org/
Show menu
Imagine a square swimming pool with 24 single tiles around it, like the one in the diagram.
Two children stand on different tiles, and hold a ribbon or ribbons across the pool.
Each child can hold one or two ribbons at a time.
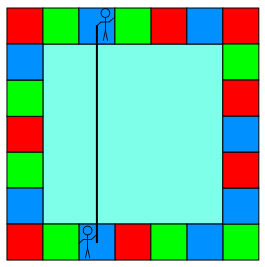
Each ribbon runs from the middle of the tile that the child is standing on.
The children are trying to make squares with their ribbons that they call 'ribbon squares'.
Here's a ribbon square they made. It has an area of 9 square tiles.
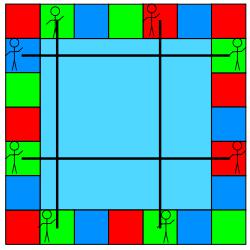
What are the smallest and largest ribbon squares they can make?
How many differently sized ribbon squares can they make?
What happens if the square swimming pool is made from 20 tiles?
How many ribbon squares can they make then?
This problem featured in a preliminary round of the Young Mathematicians' Award.
Two children stand on different tiles, and hold a ribbon or ribbons across the pool.
Each child can hold one or two ribbons at a time.

Each ribbon runs from the middle of the tile that the child is standing on.
The children are trying to make squares with their ribbons that they call 'ribbon squares'.
Here's a ribbon square they made. It has an area of 9 square tiles.

How many differently sized ribbon squares can they make?
What happens if the square swimming pool is made from 20 tiles?
How many ribbon squares can they make then?
This problem featured in a preliminary round of the Young Mathematicians' Award.