Copyright © University of Cambridge. All rights reserved.
'Indigo Interior' printed from https://nrich.maths.org/
Show menu
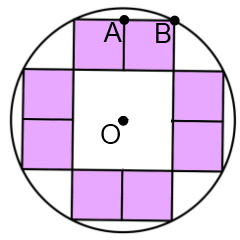 Let the centre of the circle be $O$ and let $A$ and $B$ be corners of one of the shaded squares, as shown.
Let the centre of the circle be $O$ and let $A$ and $B$ be corners of one of the shaded squares, as shown.As the circle has area $\pi$ square units, its radius is $1$ unit. So $OB$ is $1$ unit long.
Let the length of the side of each of the shaded squares be $x$ units.
By Pythagoras's Theorem, $OB^2 = OA^2 + AB^2$, that is $1^2 = (2x)^2 + x^2$.
So $5x^2=1$. Now the total shaded area is $8x^2 = 8 \times \frac{1}{5} = 1 \frac{3}{5}$ square units.
This problem is taken from the UKMT Mathematical Challenges.
You can find more short problems, arranged by curriculum topic, in our short problems collection.