 Links to the NRICH website Home page
Links to the NRICH website Home page

30 April (Primary), 1 May (Secondary)
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Seating Arrangements



- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Syed (Foxford School and
Community College, Coventry) has supplied a diagram of a
solution: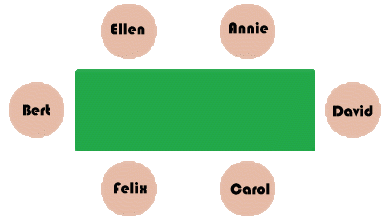
Joshua (Brooklands Primary, Suffolk) explained his thinking well:
From clue 1, two girls sit next to each other, and from clue 4, Carol did not sit beside Annie or Ellen - so Ellen and Annie must sit next to each other. From clue 5, Felix sat opposite Ellen.
So far

From clue 2, David sat opposite Bert, so I put them on the two ends of the table. Which means Carol sits next to Felix - which fits in with clue 3 (Felix sat to Carol's left).
Final answer:

Samantha (Nanyang Primary School, Singapore) realised that Bert and David could swap seats and still fit all the clues.
Josh (Ampthill, Bedfordshire) gives an excellent description:
David is sat at the left end of the table and Bert is sat facing him at the right end.Carol and Felix are sat next to each other along one side, with Carol at David's end.
Annie and Ellen are sat along the other side, with Annie at David's end so she is sat across from Carol and Allen at Bert's end so she is sat across from Felix.
Sally (Tattingstone Primary, UK) thought about the problem in a similar way to Josh.
Jason (Lark Rise Lower School, UK) was the only one to send in a different, but similar, seating arrangement:
| Carol | Felix | ||
| David | Bert | ||
| Annie | Ellen |
You may also like
Geoboards
This practical challenge invites you to investigate the different squares you can make on a square geoboard or pegboard.