This article is part of our collection Great Expectations: Probability through Problems.

How do you introduce your students to the topic of probability?

We have devised a range of problems which take a modelling approach, because we want students to be able to solve problems rather than simply calculating answers.  

We introduce two-stage problems right from the start, so that moving on from simple one-stage problems is never an issue.  As well as reducing the possibility of 'disconnect' as greater complexity is introduced, this also means we can introduce more interesting problems!  

Classes we have used this approach with have enjoyed the challenge and coped well. Take a look at this paper to read about the classroom trials we carried out.

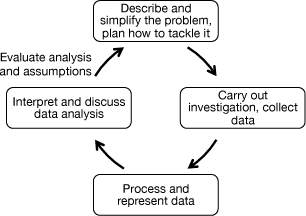The diagram on the right is the familiar modelling cycle, also known as the data-handling cycle.  Problems are structured to move students round this cycle, with some capable of exploration at more than one level.

We have based the problems on a common progression:

1. Modelling the problem practically and also in some cases through a computer simulation.  
2. Collecting data, which is then represented on a tree diagram and a 2-way table.
3. Asking questions about what the data is saying, rather than asking students to make ill-informed predictions.
4. Testing students' impressions about what the data is saying against results aggregated across the whole class.
5. Comparing experimental results with what we would expect to happen - working from a tree diagram and 2-way table completed with the expected number of each outcome at each stage, using simple probabilities (eg. chance of getting a blue face on a die with four yellow and two blue faces) to find expected results in whole numbers (natural frequencies).
6. Moving from counts to proportions.
7. Asking what the proportions will settle down to as we do more experiments.  Concept of expected results articulated.
8. Moving from expected results in whole numbers to proportions to probabilities, and then to the multiplication rule, via the tree diagram.

Fundamental in this process is the tree diagram.  The branches provide a complete set of mutually exclusive narratives, each leading uniquely to one particular outcome, with no possible outcome omitted.  

Making tree diagrams fundamental right from the start helps to avoid problems with outcomes not being properly enumerated - for instance, when flipping two coins recognising the difference between HT and TH.  

Using tree diagrams as a means to represent data also helps students to become very familiar with them, and comfortable using them, long before they need to use them to calculate probabilities.  Using whole numbers on tree diagrams and on 2-way tables helps students to become aware of the importance of identifying correctly the appropriate values for the numerator and denominator, when expressing an answer as a proportion or as a probability.

Our problems also provide a progression through the curriculum secondary students are expected to follow:

• Age 11-12 - practical experimentation as a means of collecting data, representation of the data in tabulated results, then a tally, then a tree diagram and 2-way table.  Main focus is on outcomes.
• Age 12-13 - building on this experience through further practical work and data collection, again using tree diagrams and 2-way tables.  Main focus on expected results, proportion and the significance of the denominator (ie. choosing the appropriate subset of the data).
• Age 13-15 - again, practical experimentation, data collection, representation of practical results and expected results on tree diagrams and 2-way tables, using the proportions in the expected results on the tree diagram to establish the multiplication rule.  Additional focus on sample spaces.
• Age 14-16 - again, practical experimentation and data collection, representation of results and expected results on a tree diagram, using probabilities and the multiplication rule to explore sampling with and without replacement.  Focus on independent and dependent events.