Sticky Triangles

'Sticky Triangles' printed from https://nrich.maths.org/

Show menu

Sticky Triangles

I used three lolly sticks to make one small triangle:

I added more lolly sticks to make four small triangles:

How many lolly sticks have I used altogether now?

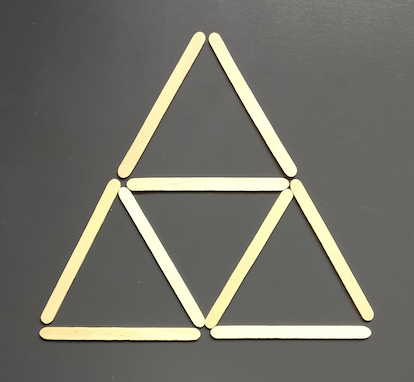
I decided to add another 'row' of small triangles.
Can you picture in your head what this will look like?
How many small triangles will there be?
How many lolly sticks will I have used in total?

Once you have had a think, you might like to check by making the pattern yourself, or by sketching it.
You could click below to see what my pattern looked like.

I carried on adding rows of small triangles, and I found many patterns.

Have a go yourself! You could use lolly sticks or straws or..., or you could draw lines.

Can you describe any patterns that you find?
Can you predict how the pattern will carry on?
Why does the pattern occur?

Will the pattern continue for ever? How do you know?

Why do this problem?

This investigation starts in a very practical way so that all learners can take part. It can lead to several interesting number patterns and is a good context in which pupils can begin to generalise.

Possible approach

You could draw one triangle on the board and indicate that it is made from three lolly sticks (or lines etc). Tell the class that you are going to extend the drawing with another row of triangles, and draw them underneath the first small triangle. Ask how many more lolly sticks/lines you have used and therefore how many are now used altogether.

Invite children to predict how many more lines will be needed for another row in the pattern. Can they make a prediction without drawing? Take some suggestions with reasons, then check how many are needed by drawing the arrangement. Focusing on the different ways the children explain how they visualised the arrangement will help them to build up a pattern of what is happening.

Set the group off on the challenge. You could leave it very open-ended or you could say, for example, that you would like them to be able to work out the total number of matches for ten rows of triangles. As they work, stop them at various intervals to share effective ways of recording results. Learners might find it helpful to have lolly sticks, and/or isometric lined or dotty paper for recording the triangles.

To encourage them to look more carefully at the number patterns involved, rather than simply counting lolly sticks each time, suggest that you would like them, for example, to be able to work out the total number of lolly sticks for 100 rows of triangles, which of course would take far too long to draw and count.

Key questions

How will you record what you have done?

Can you see why the number of lolly sticks increased by that amount when you added that row?

Can you predict how many lolly sticks the next row will need? Why?
Can you see a link between the number of rows and the total number of lolly sticks?
Can you see a link between the number of small triangles and the total number of lolly sticks?

Possible support

Some pupils will be able to organise their results without help but others might need the guidance of a table, perhaps rather like this:

Possible extension

Some learners will be able to express the patterns they have found in terms of words, whereas some might being to use algebra if they are familiar with it. The real challenge here is to explain the patterns found in the numbers.

Learners could create their own growing pattern with squares or even hexagons. Alternatively, they could have a go at Seven Squares, which encourages learners to explore structure.