Copyright © University of Cambridge. All rights reserved.
'Sweets in a Box' printed from https://nrich.maths.org/
Show menu
Gabe and Zoran from Mrs. Hovish's Math Class from the Athena Academy in the United States
At first we were confused so we started by placing beads in random places. Then we started moving the "candies" around so that they would follow the rules. Then we started seeing a pattern! We noticed that the same 2 colors were always in a row together so we just finished by following that. I ended up with 6 rows and 6 columns. Gabe had 4 rows and 9 columns. We used a multiplication chart and saw that 6X6 = 36 and 9X4= 36 too! Here are their pictures.


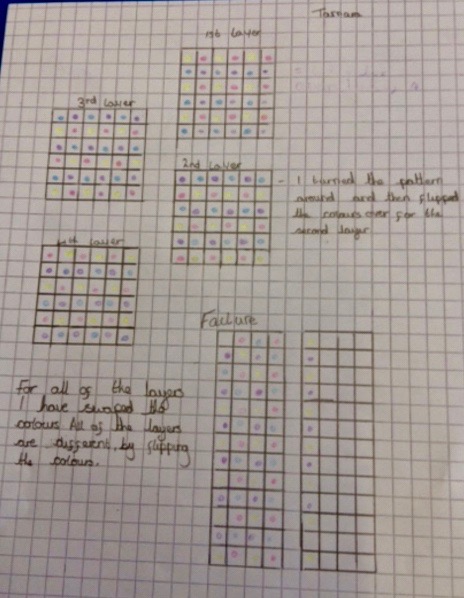
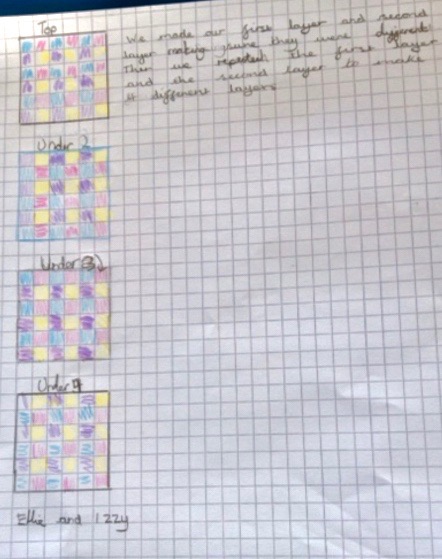
Tamara, Ellie and Izzy, Lily and Isabelle from St. Andrew's, Enfield sent in these three solutions.

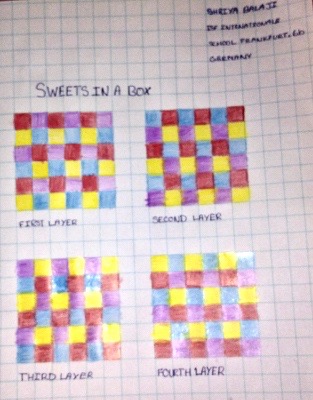
Shriya from the International School in Frankfurt Germany sent in these three excellent different designs.
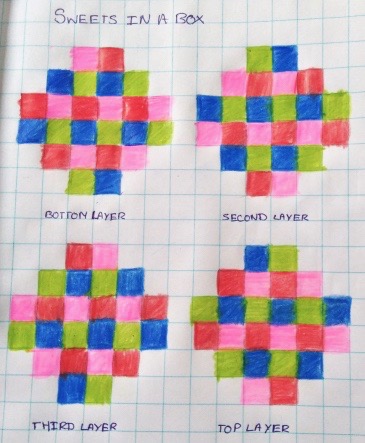
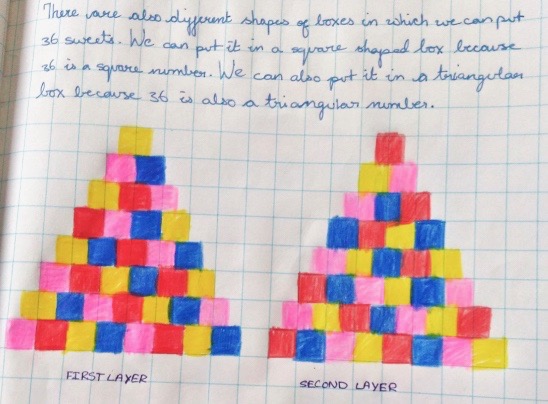
Lily and Isabelle from St.Michaels School sent in this solution:

Well these were really good solutions, well done everyone.