A continuous, differentiable curve $y = f(x)$ enters a region of the x-y plane with gradient $+1$ at the point $(1, 1)$ and emerges from the region with gradient $+1$ at either point $A=(3, 4)$, $B=(3, 3)$ or $C=(3, 2)$. Another curve $y = g(x)$ enters a region of the x-y plane with gradient $+1$ at the point $(1, 3)$ and emerges from the region with gradient $-1$ at either point $D=(3, 4)$, $E=(3, 3)$ or $F=(3, 2)$.


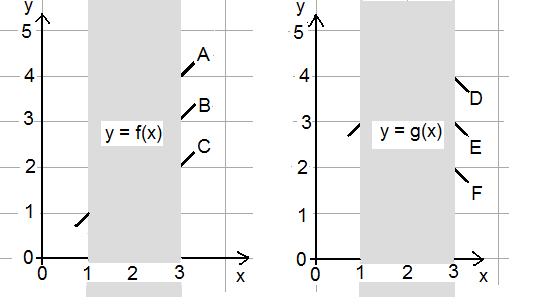
For each of the 6 possibilities, sketch - no equations required -  possible forms of the graphs of the gradients of the functions against $x$.

In which cases must the gradient function necessarily be zero at at least one place in the obscured regions?

In which cases must the gradient of the gradient function necessarily be zero at at least one place in the obscured regions? Give a clear argument.

Find equations for 6 functions which have the correct gradients at these points.
 
 

Extensions: If the gradients at points $A$ to $F$ were allowed to vary, under which circumstances might there be no zeros in the gradients and the gradients of the gradients? Explore the possibility of two different functions matching the conditions for $B$.