Well done to everybody who had a go at this problem. We received an anonymous solution which said:

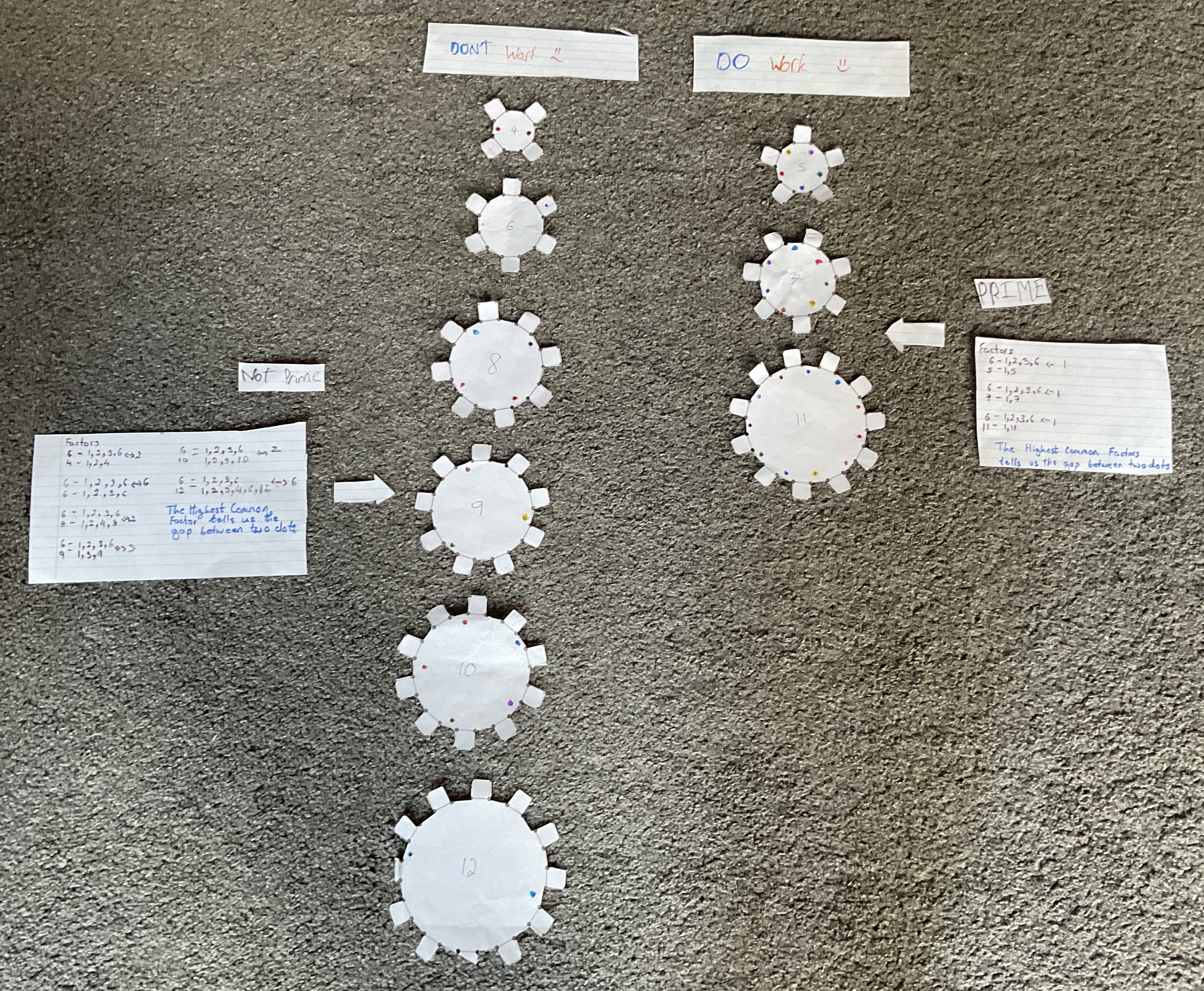
I notice that the space between the dots is the same number as the smaller cog. (For example, pairing the 12-cog with the 4-cog means that the marked tooth on the 4-cog goes into every fourth gap in the 12-cog.) So I have to find a number that the dots will be next to each other. 

12 and 4 don't work but 5 and 6 work. I realised that 6-5=1, so the difference is one!

I start trying to prove this. 8-7=1 so I thought it would work. And I was right.

I decided to test some numbers that I predicted that won't work. 11-9=2. But it did!

This is very interesting. We received quite a few solutions from children who thought that two cogs with consecutive numbers would lead to the coloured tooth going into every 'gap', and those children often suggested that this wouldn't happen with two cogs with non-consecutive numbers. But as you've noticed, the 11-cog and the 9-cog do work! I wonder if there's something else going on with the numbers?

Dhruv from The Glasgow Academy in the UK looked at which cogs would let the the coloured tooth go into every gap when paired with the cog with six teeth. This picture can be clicked on to make it bigger:

Dhruv looked at the factors of the number of teeth of each cog, and noticed that the cogs that don't work when paired with the 6-cog share some factors (other than 1) with 6. This is an interesting observation - I wonder why this means that the coloured tooth won't go into every gap?

Shaunak from Ganit Manthan, Vicharvatika in India sent in this explanation and video:

Which pairs of cogs let the coloured tooth go into every 'gap' on the other cog?

If the numbers represent the number of teeth/gaps on each cog with the following notation, for example, the pairs in which the cogs let the coloured tooth of the first cog go into every gap on the other cog are:

(4, 5), (4, 7), (4, 9), (4, 11), (5, 6), (5, 7), (5, 8), (5, 9), (5, 11), (5, 12), (6, 7), (6, 11), (7, 8), (7, 9), (7, 10), (7, 11), (7, 12), (8, 9), (8, 11), (9, 10), (9, 11), (10, 11), (11, 12).

Which pairs do not let this happen? Why?

The pairs which will not work are:

(4, 4), (4, 6), (4, 8), (4, 10), (4, 12), (5, 10), (6, 6), (6, 8), (6, 9), (6, 10), (6, 12), (8, 8), (8, 10), (8, 12), (9, 9), (9, 12), (10, 10), (10, 12), (11, 11), (12, 12).

These pairs will not work because the tooth of the first cog touches only spots after a specific interval. This interval is the number of teeth on the first cog. If the HGF (highest common factor - the largest number that is a factor of both numbers) of the number of teeth on the first cog and the number of gaps on the second cog is x, then the coloured tooth will touch x gaps on the other cog.

If x = 1, then the coloured tooth will touch all gaps on the other cog.

This looks good, Shaunak - I think there is just one more pair that won't work, where both cogs have the same number of teeth.

Can you explain how to determine which pairs will work, and why?

The technique to figure out if a pair will work or not is as follows:

First, count the number of teeth on the first cog and the number of gaps on the second cog.

Next, find their HCF. If the HCF is 1, then the tooth will go on every other gap, else the pair will not work.

These are some good ideas, Shaunak! Thank you for sharing your method with us.

Thank you as well to Ahana, Sehar, Saanvi, Dhanvin, Aariz, Ananthjith, Vivaan, Sai, Pranathi, Paavani, Utkarsh and Dhruv from Ganit Kreeda, Vicharvatika in India, who all worked very hard on this problem. Take a look at Ganit Kreeda's full solution to see their ideas - they used the fact that the teeth on a cog go up to a certain number and then restart, like the numbers on a clock face do, to help them solve this problem.