Steve said the following

I sketched four vertical asymptotes and a sketch showed that a function which decayed to zero from above at $x \rightarrow \pm \infty$ could have the right sorts of properties.

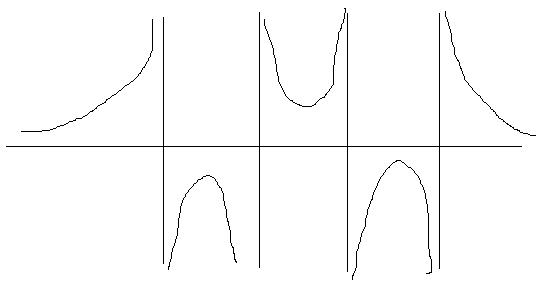
To get the right asymptotes and behaviour at $\pm \infty$ I guessed the following curve, choosing to make it symmetric about the origin for simplicity
$$
y = \frac{1}{(x-2)(x-1)(x+1)(x+2)}
$$
This worked: it has a turning point at $x$ between $-2$ and $-1$ another turning point at $x$ between $1$ and $2$ and a turning point at $x=0$.

The plot of this from graphmatica is as follows



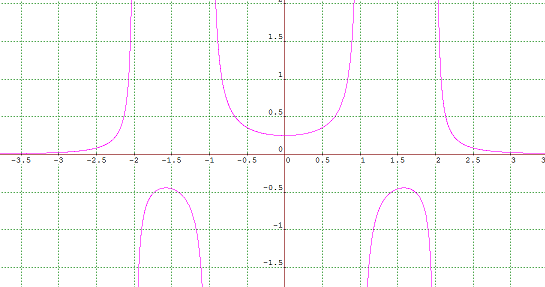
It seems likely that many such curves, with differing constants, would also give the correct behaviour. To see why, upon differentiation, I get a cubic polynomial divided by another polynomial. For zeros the numerator would need to be zero and a cubic can have three real roots. I could choose the constants to have the correct number of real roots.

I then considered the second request. Initially, I thought that this seemed impossible, but then started to work through the possibilities for asymptotes. By turning the middle turning point into a point of inflection I would have a graph with the correct behaviour.


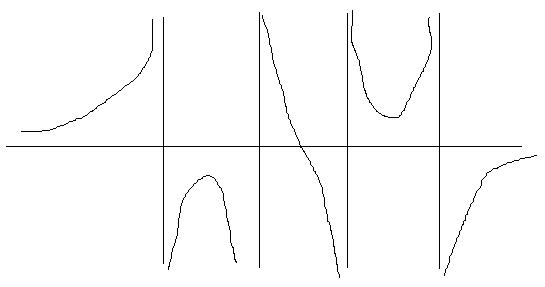
I wondered how to convert the behaviour of the central turning point and decided that the curve needed to be forced to pass through the origin and also to be antisymmetric. I therefore multiplied the expression by $x$, realising that this wouldn't affect the 'topological' behaviour at the other turning points. A plot of the curve
$$
y = \frac{x}{(x-2)(x-1)(x+1)(x+2)}
$$
gave graph



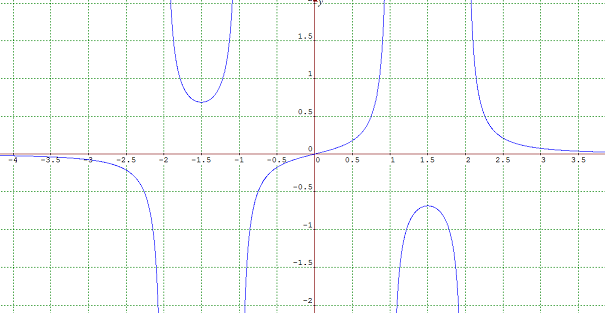
Which has the correct behaviour.