Copyright © University of Cambridge. All rights reserved.
'Symmetric Angles' printed from https://nrich.maths.org/
Show menu
As the figure has rotational symmetry of order $4$, $ABEF$ is a square.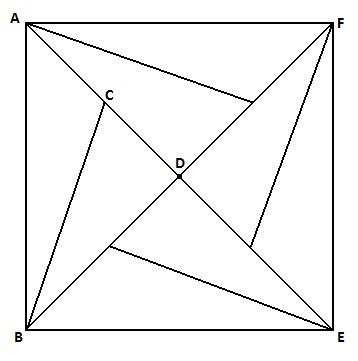
Area $ABEF=4\times$area$\triangle BDA=4\times \frac{1}{2}BD \times DA=2(DB)^2=24$cm$^2$, so $BD=\sqrt{12}$cm$=2\sqrt{3}$cm.
As $ABEF$ is a square, $\angle ABD=45^{\circ}$ so $\angle CBD=45^{\circ} -15^{\circ} =30^{\circ}$.
Since $\tan {30^{\circ}}= \frac{CD}{BD}=\frac{CD}{2\sqrt{3}}$, we have $CD=2\sqrt{3}\tan{30^{\circ}}$cm.
Now consider the following equilateral triangle with side lengths $2$:
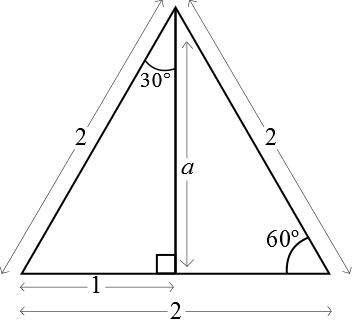
The vertical line is perpendicular to the base-line and so bisects both the angle at the top vertex and the base-line.
Consider the left right-angled triangle. Pythagoras' theorem gives $a=\sqrt{3}$ and then we have $\tan{30^{\circ}}=\frac{1}{a}=\frac{1}{\sqrt{3}}$
Therefore $CD=2\sqrt{3}\tan{30^{\circ}}$cm$=2$cm.

Area $ABEF=4\times$area$\triangle BDA=4\times \frac{1}{2}BD \times DA=2(DB)^2=24$cm$^2$, so $BD=\sqrt{12}$cm$=2\sqrt{3}$cm.
As $ABEF$ is a square, $\angle ABD=45^{\circ}$ so $\angle CBD=45^{\circ} -15^{\circ} =30^{\circ}$.
Since $\tan {30^{\circ}}= \frac{CD}{BD}=\frac{CD}{2\sqrt{3}}$, we have $CD=2\sqrt{3}\tan{30^{\circ}}$cm.
Now consider the following equilateral triangle with side lengths $2$:

The vertical line is perpendicular to the base-line and so bisects both the angle at the top vertex and the base-line.
Consider the left right-angled triangle. Pythagoras' theorem gives $a=\sqrt{3}$ and then we have $\tan{30^{\circ}}=\frac{1}{a}=\frac{1}{\sqrt{3}}$
Therefore $CD=2\sqrt{3}\tan{30^{\circ}}$cm$=2$cm.
This problem is taken from the UKMT Mathematical Challenges.
You can find more short problems, arranged by curriculum topic, in our short problems collection.