Links to the University of Cambridge website
 Links to the NRICH website Home page
Links to the NRICH website Home page

 Links to the NRICH website Home page
Links to the NRICH website Home page

Nurturing young mathematicians: teacher webinars
30 April (Primary), 1 May (Secondary)
30 April (Primary), 1 May (Secondary)
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Star Product Sudoku
Age 11 to 16
Challenge Level 





By Henry Kwok
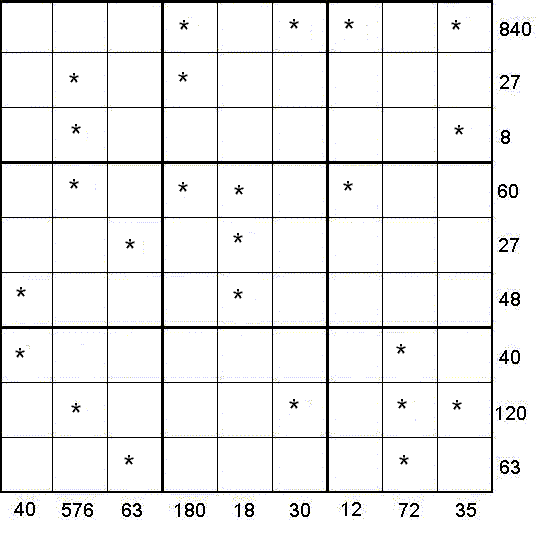
Rules of Multiplication Equation Sudoku
Like the standard sudoku, this sudoku variant has two basic
rules:
- Each column, each row and each box ($3\times3$ subgrid) must have the numbers $1$ through $9$.
- No column, row or box can have two squares with the same number.
The puzzle can be solved by finding the values of the unknown
digits (all indicated by asterisks) in the squares of the
$9\times9$ grid. At the bottom and right side of the $9\times9$
grid are numbers, each of which is the product of a column or row
of unknown digits marked by asterisks. Altogether a set of 18
equations can be formed from the columns and rows of unknown digits
and constants.
For example, in the first and second columns beginning from
the left of the $9\times9$ grid, the following equations can be
formed:
$ *\times* = 40$
and
$*\times*\times*\times* = 576$.
In the fourth and fifth rows beginning from the top of the
$9\times9$ grid, the following equations can be formed:
$*\times*\times*\times* = 60$
and
$*\times* = 27$.
After solving all the equations, the puzzle is solved by the
usual sudoku technique and strategy.