The best solution to this problem came from Andrei Lazanu, age 13 of School No. 205, Bucharest, Romania. Can you finish off the solution?

I drew a right angled triangle, and circles having as centres the vertices of the triangle, and being tangent two by two at points on the sides of the triangle.

Let $r_A,\ r_B$ and $r_C$ be the radii of the touching circles centred at the vertices of the right angled triangle ABC, of sides $a,\ b$ and $c$, with the centres at the points A, B and C respectively. These radii satisfy the conditions:

$$\eqalign{ r_A + r_B &= c \cr r_B + r_C &= a \cr r_C + r_A &= b.}$$

This system of three equations with three unknowns could be solved in the following manner: adding all three equations, dividing the result by two and subtracting one by one the equations. I obtained:

$$\eqalign{ r_A &= {1\over 2}(b+c-a) \cr r_B &= {1\over 2}(a + c - b) \cr r_C &= {1\over 2}(a + b - c) \cr.}$$

I also drew the three escribed circles, as well as their points of intersection. I see that on each side, the points of intersection of the circles with centres at the vertices are the same as the points of tangency of the escribed circles with the sides. This can be explained by the fact that the lengths of the tangents to a circle, drawn from a point exterior to the circle, have equal lengths. This will enable us to find the radius of an escribed circle.

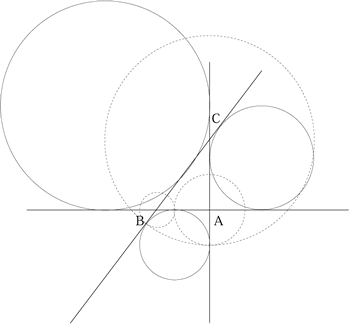
Given any right angled triangle $ABC$ with sides $a,\ b$ and $c$, we have to find the radii of the three circles shown in the diagram with centres at $A,\ B$ and $C$ such that each circle touches the other two and two of the circles touch on $AB$ between $A$ and $B$, two circles touch on $CA$ produced and two circles touch on $CB$ produced.

The method is exactly as above but here we have

$$\eqalign{r_A + r_B &= c \cr r_C - r_A &= b\cr r_C - r_B &= a.}$$

By solving these equations and identifying which circles have equal radii you should be able to find the radius of one of the escribed circles.

The radii of the other escribed circles are found similarly.