Copyright © University of Cambridge. All rights reserved.
'Look Before You Leap' printed from https://nrich.maths.org/
Show menu
The diagonals of a square meet at $O$.
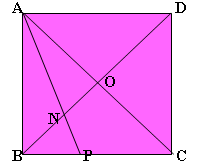
The bisector of angle $OAB$ meets $BO$ and $BC$ at $N$ and $P$ respectively.
The length of $NO$ is $24$.
How long is $PC$?