(a) Prove that a triangle with sides $3$, $7$, $8$ contains a $60$ degree angle.

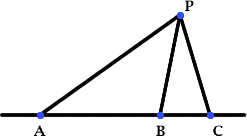
(b) Three points $A$, $B$ and $C$ lie in this order on a line, and $P$ is any point in the plane. Use the Cosine Rule to prove that:

\[{AP^2\over AB.AC}+{CP^2\over CA.CB} = 1 + {PB^2\over BA.BC}.\]