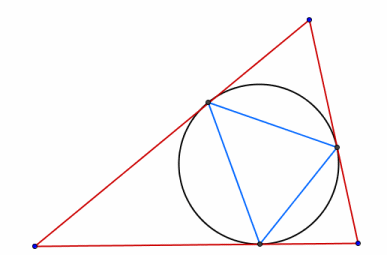
Start with any triangle. Draw its inscribed circle (the circle which just touches each side of the triangle). Draw the triangle which has its vertices at the points of contact between your original triangle and its incircle. Now keep repeating this process starting with the new triangle to form a sequence of nested triangles and circles. What happens to the triangles?
 

If the angles in the first triangle are $a$, $b$ and $c$ prove that the angles in the second triangle are given (in degrees) by

$f(x) = (90 - x/2)$

where $x$ takes the values $a$, $b$ and $c$. Choose some triangles, investigate this iteration numerically and try to give reasons for what happens.

Investigate what happens if you reverse this process (triangle to circumcircle to triangle...)