Copyright © University of Cambridge. All rights reserved.
'Set Square' printed from https://nrich.maths.org/
Show menu
The locus of the point $P$ is a straight line as the vertices $Q$ and $R$ slide along the walls.
 |
Sue Liu of Madras
College, St Andrew's sent this solution to the problem. Without
loss of generality we can let the length of $QR$ be 1 unit, and
take a coordinate system with the origin at $O$ and axes along $OR$
and $OQ$.
If $\angle PQR = \alpha$, where $0 < \alpha < 90^\circ$,
then $PQ = \cos \alpha$ and $PR = \sin \alpha.$ Let $\angle QRO =
\theta $ where $0 \leq \theta \leq 90^\circ$. Then, from the right
angled triangles $PSQ$ and $PTR$, we have $\angle PRT = \angle QPS
= \alpha - \theta$, and hence we can write down the coordinates of
the point $P$.
|
\begin{eqnarray} x &=& \cos \alpha \cos
(\alpha - \theta) \\ y &=& \sin \alpha \cos (\alpha -
\theta). \end{eqnarray}
We see that $${y\over x}= {\sin \alpha \cos (\alpha -
\theta)\over \cos \alpha \cos (\alpha - \theta)}= \tan \alpha.$$
and so $P$ lies on the straight line $y = x\tan \alpha$.
The position $(x,y)$ depends only on $cos(\alpha - \theta)$,
$\alpha$ being a constant, and $\theta$ a variable. The distance of
the point $P$ from $O$ is given by $$OP^2 = x^2 + y^2 =
\cos^2(\alpha - \theta)(\cos^2\alpha + \sin^2\alpha) =
\cos^2(\alpha - \theta).$$ Hence $OP = \cos(\alpha - \theta)$ which
is a maximum when $\cos(\alpha - \theta) = 1$, that is when $\alpha
= \theta$. This occurs when $OQPR$ is a rectangle as shown in the
diagram.
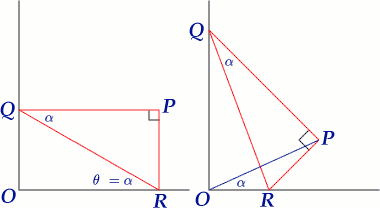
We get an even simpler method of solution by using the fact that the angles $QOR$ and $QPR$ are both 90 degrees so that $OQPR$ is a cyclic quadrilateral with $PR$ as a chord. We have $\angle POR = \angle PQR = \alpha$ because these two angles are subtended by the same chord of the circle. This shows that $\angle POR$ is constant and hence that the locus of $P$ is the straight line $y = x \tan \alpha.$
What can you say about the locus of $P$ if the triangle $PQR$ is not a right angled triangle?