Rots and Refs

'Rots and Refs' printed from https://nrich.maths.org/

Show menu

The point $P$ has coordinates $(r\cos \phi, r\sin \phi)$ where the distance of $P$ from the origin is $OP=r$ and the line $OP$ is at angle $\phi$ to the x axis. Find the image of this point under the transformation given by the matrix

$\mathbf{T_1}=\left( \begin{array}{cc} \cos \theta & -\sin \theta\\ \sin \theta & \cos \theta \end{array} \right)$

Draw a diagram and describe the effect of this transformation on the points of the plane.

The point $P$ has coordinates $(p,q)$ and the point $P'$ is the reflection of $P$ in the line $y = x\tan\theta$. In the diagram below the lines $P'X'$ and $XA'$ are perpendicular to the line $OA'X'$. "

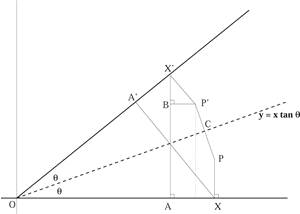
Reflection

Prove that $OX = OX' = p$, $P'X' = PX = q$ and $OA = OA' = p\cos 2\theta.$ Find the lengths $BP',\ AX'$ and $BX'$ and hence prove that transformation given by the matrix

$\mathbf{T_2}=\left( \begin{array}{cc} \cos 2\theta & \sin2\theta\\ \sin2\theta & -\cos2\theta \end{array} \right)$

gives a reflection in the line $y=\tan\theta$.