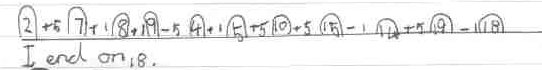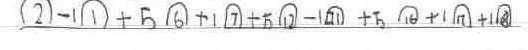Copyright © University of Cambridge. All rights reserved.
Fiona from Tattingstone School tackled this
very clearly:
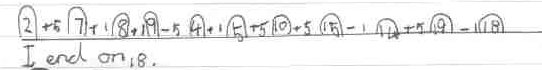
She found another way of starting and ending
on these numbers:
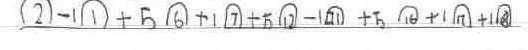
Fiona then explains:

Omar from the Modern English School, Cairo
drew out a few different routes which also start at $2$ and end at
$18$:
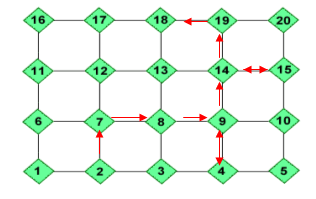
I like the way you've shown the 'optional
extras' with double-headed arrows, Omar Abdel
also from the Modern English School found
another route:
$2+1+1+5-5+1+5+5-1+5-1$
Elliot, Richard and Christopher from Moorfield
Junior School agreed with Fiona but also found another equally
short route: $+1,+5,+5,+5$.
Molly and Callum from Bradon Forest School
sent us a detailed response:
The last number in the sequence is $18$, and another Sequence is
$2(+5)7(+5)12(+5)17(+1)18$.
But the last one is the hardest. Still using the example
$2$-$18$ above you need to subtract the biggest number from the
smallest one ($18-2=16$)
The number you are left with is the number that all the steps
shold add up to, or 'special number' ($5+5+5+1=16$) or
($5+1+1-5+1+5+5-1+5-1=16$) To find out the number of steps and what
they are add keep adding together $5$'s until you reach the number
closest to the special number ($3\times5=15$ is closer to $16$ than
$4\times5=20$). Then continue adding or subtracting $1$'s until you
get the special number ($15+(1\times1)=16$). This might be a bit
clearer;
$3\times5 = 15$
$1\times1 = 01$ = $16$ --special number in four steps
You can try that on any sequence and it'll still work!!!
Luke from Witton Middle School noticed
something important:
For the first part of the challenge, I worked out that the
number you ended on was $1$8.
So for the second part of the challenge, I did:
$2 +1 (3) +5 (8) -1 (7) +5 (12) -1 (11) +5 (16) +1 (17) -5
(12) +1 (13) +5 =18$
Then I used cancelling down (for example, $+1$ and $-1$ can be
cancelled out because whatever number is the input, the output will
be the same as the input. e.g. $32 +1 -1 =32$).
To end up with the shortest route - $2 +5 +5 +5 +1 =18$.
You could add in a different order but the answer would be the
same.
Well done, Luke, you're right that the order
of the operations is not important - you would still get to
$18$.