How long is the Cantor Set?
Take a line segment of length 1. Remove the middle third. Remove
the middle thirds of what you have left. Repeat infinitely many
times, and you have the Cantor Set. Can you find its length?
Age
11 to 14
Challenge level
Problem
In the problem The Cantor Set, we met the Cantor set, which is the limit of $C_n$ as $n$ tends to infinity.
Image
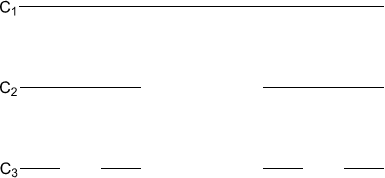
We can talk about the length of one of our sets $C_n$.
The set $C_1$ has length 1.
The set $C_2$ has length $\frac{2}{3}$, as this is the total length of the line segments in $C_2$.
What are the lengths of $C_3$, $C_4$ and $C_5$?
Can you find a general expression for the length of $C_n$?
By considering what happens as $n$ tends to infinity, can you find the length of the Cantor set?
Getting Started
If you haven't tried it already, you are strongly encouraged to tackle the problem The Cantor Set before you try this problem.
Student Solutions
Gregory from Magnus C of E School in Nottingham and Luke from St Patrick's School reasoned correctly. Here is Luke's explanation:
For this question I found a pattern in it:
For example $C_1$ has length of 1 and $C_2$ has length of
$\frac{2}{3}$
and there is a pattern in this because 1 $\times$2 = 2, so
there is your numerator,
and to get your denominator you multiply 1 by 3, so there you
have your $\frac{2}{3}$.
So to put this into easier words, it simply means multiply the
numerator by 2 the whole way and multiply the denominator by
3.
So there is my strategy.
So the answer to $C_3$ is $\frac{4}{9}$
and the answer to $C_4$ is $\frac{8}{27}$
and finally $C_5$ is $\frac{16}{81}$.
$C_n$ is $\left(\frac{2}{3}\right)^{n-1}$
and when n $\rightarrow$ infinity, $ C_n \rightarrow$ 0
David from Gordonstoun School got the same
result and added:
In a geometric progression,
$a$ = $ 1^{st}$ term
$r$ = constant factor
$n$ = number of terms
Any value in a geometric progression is $ ar^{n-1}$
In this case
$a$ = 1
$r$ = $\frac{2}{3}$
$r$ = $\frac{2}{3}$ (which is less than 1),
so the higher its power, the closer the result is to
zero.
(Any positive number smaller than 1, to the power of infinity,
tends to zero.
Therefore $ r^{n}$ tends to zero)
So as $n$ tends to infinity,
$ar^{n-1}$ tends to $a \times 0 = 0$
So the Cantor Set's length is zero.
Liam from Wilbarston School reasoned in a
similar way:
The length of $C_{n+1}$ is simply two thirds of the length of $C_n$,
as $C_{n+1}$ is purely $C_n$ with the middle thirds removed.
Now taking $L_n$ to be the length of $C_n$:
$L_2$ = $\frac{2}{3}$,
$L_3$ = $\frac{4}{9}$,
$L_4$ = $\frac{8}{27}$ etc. etc.
It's obvious that $L_n$ = $\left(\frac{2}{3}\right)^{n-1}$.
So as n tends to infinity, $L_n$ gets increasingly smaller, i.e. tends to zero.
Therefore the length of the Cantor set is zero. In fact, the Cantor set is a set of points, because endpoints of line segments will never be removed, only middle thirds.
And as Euclid said, 'A point is that which has no part', i.e. a point has zero length, zero width and zero height.
Well done to you all.
Teachers' Resources
See the Notes for the problem The Cantor Set .
The Cantor set is also an example of a mathematical object called a fractal . Fractals and some of their properties are explored in the NRICH problems Squareflake , Sierpinski Triangle and Von Koch Curve .