Where are the primes?
Problem
Here are some prime numbers:
$5, 17, 23, 59, 89, 101$
They are all odd. What else do they have in common?
Here are some more prime numbers:
$13, 19, 31, 37, 61, 67$
They are also all odd. What else do they have in common?
Can you find any primes greater than $3$ which are not one more, or one less, than a multiple of $6$?
Charlie thought that it wasn't possible to find any primes which were not one more, or one less than a mutiple of $6$. He thought that he might be able to use a number grid to help him prove this.
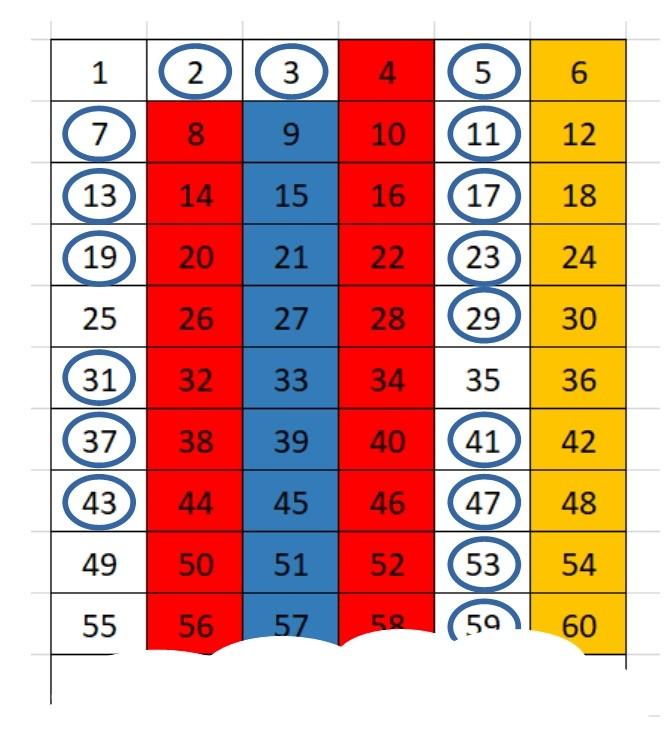
Why do you think some numbers are shaded in red, blue or yellow?
What do you notice about the numbers in circles?
Claire also though that it wasn't possible to find any primes which were not one more, or one less than a mutiple of $6$. She thought that she might be able to use some general expressions to help her prove this.
We know that $6n$ is a multiple of $6$, so cannot be prime.
We can write $6n+2=2(3n+1)$, and so we know that $6n+2$ is a multiple of $2$. The only multiple of $2$ which is prime is $2$ itself, which is not greater than $3$.
Can you complete Claire's method to show that primes numbers greater than $3$ must be of the form $6n+1$ or $6n+5$?
Can you show that you can write $6n+5$ in the form $6m-1$, and so show that numbers of the form $6n+5$ are one less than a multiple of $6$?
Student Solutions
Jayanth from GESS in Singapore noticed what linked the prime numbers:

Here, the difference of any 2 numbers is a multiple of 6. In both the hint & the answer, it’s like that. 6, 12, 30 & 48 are all multiples of 6.
They all have a difference of a multiple of 6 when 2 numbers are subtracted with each other (bigger number subtracting a smaller number, not the other way around).
From comparing the two lists, you can also see that these prime numbers are all one more than a multiple of 6. The prime numbers on the first list are all one less than a multiple of 6.
Yazan from Dubai International Academy in Jordan sent in some great ideas. Yazan uses the word 'composite' to mean 'not prime'.
I don’t think there is a prime greater than 3 which is not one greater or one less than six.
Clarie’s method shows that. For example 13 is a prime number, however, it is $6n+1,$ which means it is one greater than a multiple of six, and 103 is also prime, however it is $6n+5,$ or $6n-1,$ which means it is one less than a multiple of six. All of the primes greater than three are like that.
$6n+3$ is neither one greater nor one less than a multiple of six, but it is a multiple of 3, so they’re composite, except for three which is prime (factors of 3: 1 and 3 only), but we are looking than primes greater than 3.
To conclude, I couldn’t find a prime greater than 3 which is neither one more or one less than a multiple of six.
Sunhari from British School Muscat completed Claire's proof. Sunhari uses $|$ to mean 'is a multiple of' (for example, $3|6$ is another way of writing '3 is a multiple of 6').
$6n+1$ cannot be factorised further, and is therefore a prime.
Note here that $6n+1$ doesn't have to be a prime - for example, if $n=4$
$6n+3 = 3(2n+1)$
Therefore $3|6n+3,$ and $6n+3$ is composite.
$6n+4 = 2(3n+2)$
Therefore $2|6n+4$ and $6n+4$ is composite.
$6n+5$ cannot be factorised, and is therefore a prime.
Note again that $6n+5$ can be prime but doesn't have to be prime - for example, if $n=5$
Suppose $a = 6n+5$
$a + 6 = 6n+5+6$
$a+6 = 6n +6 +5$
$a+6 = 6(n+1) +5$
$a+6 = 6m +5 for $m = n+1$
$a = 6m +5-6$
$a= 6m-1$
Therefore, all primes over 3 can be written as one less or one more than a multiple of 6. (Since any numbers that are not one less or one more than a multiple of 6 are composite.)
Teachers' Resources
Why do this problem?
This problem, along with the rest of the problems in the Proof for All (st)ages feature, provides an excellent context for observing, conjecturing and thinking about proof, and for appreciating the power of algebra.
Possible support
Encourage students to work in pairs and test out their ideas.
